11. Comparing Proportions
11.1 z-Test for Comparing Proportions
In Section 9.4 we covered a one-sample test for proportions using the ![]() approximation to the binomial distribution. Here we want to compare a proportion
approximation to the binomial distribution. Here we want to compare a proportion ![]() in one population with
in one population with ![]() in another population, a two-sample test for proportions, also using the
in another population, a two-sample test for proportions, also using the ![]() approximation to the binomial distribution. Define
approximation to the binomial distribution. Define
![]()
where ![]() and
and ![]() are the number of items of interest in the samples from the two populations and
are the number of items of interest in the samples from the two populations and ![]() and
and ![]() are their sample sizes. Also define the corresponding
are their sample sizes. Also define the corresponding ![]() ,
, ![]() ,
, ![]() and
and ![]() . The hypotheses we want to test is
. The hypotheses we want to test is
![]()
which is equivalent to
![]()
If ![]() , and
, and ![]() are all
are all ![]() then the appropriate normal distribution will provide a good approximation to the relevant binomial distribution and we can use the following test statistic to test the hypotheses
then the appropriate normal distribution will provide a good approximation to the relevant binomial distribution and we can use the following test statistic to test the hypotheses
![Rendered by QuickLaTeX.com \[ z_{\rm test} = \frac{\hat{p}_{1} - \hat{p}_{2}}{\sqrt{\bar{p}\bar{q} \left( \frac{1}{n_{1}} + \frac{1}{n_{2}} \right)}} \]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-dc1f3e4de198ebd05245547504e9e99a_l3.png)
where
![]()
are the proportions of items of interest and not of interest in the two samples combined.
Example 11.1 : In a nursing home study we are interested in the proportions of nursing homes that have vaccination rates of less than 80![]() . The two populations we want to compare are small nursing homes and large nursing homes. In a sample of 34 small nursing homes, 12 were found to have a vaccination rate of less than 80
. The two populations we want to compare are small nursing homes and large nursing homes. In a sample of 34 small nursing homes, 12 were found to have a vaccination rate of less than 80![]() . In a sample of 24 large nursing homes, 17 were found to have a vaccination rate of less than 80
. In a sample of 24 large nursing homes, 17 were found to have a vaccination rate of less than 80![]() . At
. At ![]() is there a difference in the proportions of small and large nursing homes with vaccination rates of less than 80
is there a difference in the proportions of small and large nursing homes with vaccination rates of less than 80![]() ?
?
Solution :
0. Data reduction.
First define: population 1 = small nursing homes and population 2 = large nursing homes. Then compute the proportions:
![]()
![]()
1. Hypotheses.
![]()
2. Critical statistic.
Use Table F, the last (![]() ) line in the column for a two-tailed test at
) line in the column for a two-tailed test at ![]() :
: ![]()
3. Test statistic.
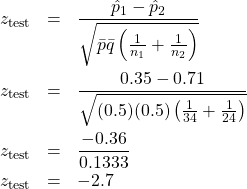
4. Decision.
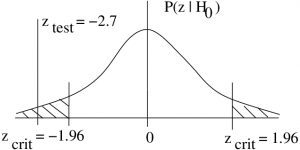
Reject ![]() .
.
5. Interpretation.
There is enough evidence, from a ![]() proportions test at
proportions test at ![]() to support the observation that large nursing homes have worse vaccination rates than small nursing homes. Make sure your parents end up in a small nursing home. (Note that rejection of
to support the observation that large nursing homes have worse vaccination rates than small nursing homes. Make sure your parents end up in a small nursing home. (Note that rejection of ![]() in a one-tail test allows us to believe the direction of difference given by the sample data.)
in a one-tail test allows us to believe the direction of difference given by the sample data.)
▢

