Chapter 11 Review
Key Terms
henry (H)
unit of inductance, ![]() ; it is also expressed as a volt second per ampere
; it is also expressed as a volt second per ampere
inductance
property of a device that tells how effectively it induces an emf in another device
inductive time constant
denoted by ![]() , the characteristic time given by quantity
, the characteristic time given by quantity ![]() of a particular series
of a particular series ![]() circuit
circuit
inductor
part of an electrical circuit to provide self-inductance, which is symbolized by a coil of wire
LC circuit
circuit composed of an ac source, inductor, and capacitor
magnetic energy density
energy stored per volume in a magnetic field
mutual inductance
geometric quantity that expresses how effective two devices are at inducing emfs in one another
RLC circuit
circuit with an ac source, resistor, inductor, and capacitor all in series.
self-inductance
effect of the device inducing emf in itself
Key Equations
| Mutual inductance by flux | |
| Mutual inductance in circuits | |
| Self-inductance in terms of magnetic flux | |
| Self-inductance in terms of emf | |
| Self-inductance of a solenoid | |
| Self-inductance of a toroid | |
| Energy stored in an inductor | |
| Current as a function of time for a |
|
| Time constant for a |
|
| Charge oscillation in |
|
| Angular frequency in |
|
| Current oscillations in |
|
| Charge as a function of time in |
|
| Angular frequency in |
Summary
11.1 Mutual Inductance
- Inductance is the property of a device that expresses how effectively it induces an emf in another device.
- Mutual inductance is the effect of two devices inducing emfs in each other.
- A change in current
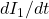 in one circuit induces an emf
in one circuit induces an emf  in the second:
in the second:
![Rendered by QuickLaTeX.com \[\mathcal{E}_2=-M\frac{dI_1}{dt},\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-d56a12228899bae076ee4728966d4056_l3.png)
where
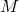 is defined to be the mutual inductance between the two circuits and the minus sign is due to Lenz’s law.
is defined to be the mutual inductance between the two circuits and the minus sign is due to Lenz’s law. - Symmetrically, a change in current
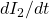 through the second circuit induces an emf
through the second circuit induces an emf 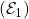 in the first:
in the first:
![Rendered by QuickLaTeX.com \[\mathcal{E}_1=-M\frac{dI_2}{dt},\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-5e733afe3e23c87a4f38bc24c22a78d3_l3.png)
where
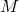 is the same mutual inductance as in the reverse process.
is the same mutual inductance as in the reverse process.
11.2 Self-Inductance and Inductors
- Current changes in a device induce an emf in the device itself, called self-inductance,
![Rendered by QuickLaTeX.com \[\mathcal{E}=-L\frac{dI}{dt},\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-d8cf1ce209a0ff959df955ae39b4a775_l3.png)
where
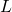 is the self-inductance of the inductor and
is the self-inductance of the inductor and 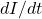 is the rate of change of current through it. The minus sign indicates that emf opposes the change in current, as required by Lenz’s law. The unit of self-inductance and inductance is the henry (
is the rate of change of current through it. The minus sign indicates that emf opposes the change in current, as required by Lenz’s law. The unit of self-inductance and inductance is the henry ( ), where
), where 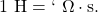
- The self-inductance of a solenoid is
![Rendered by QuickLaTeX.com \[L=\frac{\mu_0N^2A}{l},\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-b818d9422a95d6b04637a141ac83df33_l3.png)
where
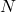 is its number of turns in the solenoid,
is its number of turns in the solenoid, 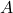 is its cross-sectional area,
is its cross-sectional area, 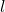 is its length, and
is its length, and 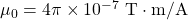 is the permeability of free space.
is the permeability of free space. - The self-inductance of a toroid is
![Rendered by QuickLaTeX.com \[L=\frac{\mu_0N^2h}{2\pi}\ln\frac{R_2}{R_1},\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-4d87286a744b5e75d88854d2cec05316_l3.png)
where
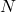 is its number of turns in the toroid,
is its number of turns in the toroid,  and
and 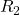 are the inner and outer radii of the toroid,
are the inner and outer radii of the toroid, 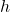 is the height of the toroid, and
is the height of the toroid, and 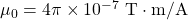 is the permeability of free space.
is the permeability of free space.
11.3 Energy in a Magnetic Field
- The energy stored in an inductor
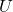 is
is
![Rendered by QuickLaTeX.com \[U=\frac{1}{2}LI^2.\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-779ad69619de74d88685e5ed5cf69f6e_l3.png)
- The self-inductance per unit length of coaxial cable is
![Rendered by QuickLaTeX.com \[\frac{L}{l}=\frac{\mu_0}{2\pi}\ln\frac{R_2}{R_1}.\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-7119bb3eecf76fbb5e1715f28ae62807_l3.png)
11.4 RL Circuits
- When a series connection of a resistor and an inductor—an
 circuit—is connected to a voltage source, the time variation of the current is
circuit—is connected to a voltage source, the time variation of the current is
![Rendered by QuickLaTeX.com \[I(t)=\frac{\mathcal{E}}{R}(1-e^{-Rt/L})=\frac{\mathcal{E}}{R}(1-e^{-t/\tau_L})~~~(\mathrm{turning~on})\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-6b8536b2f5d806a3cb50b2ff07861be9_l3.png)
(turning on),where the initial current is
- The characteristic time constant
 is
is 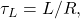 where
where 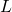 is the inductance and
is the inductance and 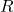 is the resistance.
is the resistance. - In the first time constant
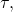 the current rises from zero to
the current rises from zero to  and to
and to 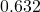 of the remainder in every subsequent time interval
of the remainder in every subsequent time interval 
- When the inductor is shorted through a resistor, current decreases as
![Rendered by QuickLaTeX.com \[I(t)=\frac{\mathcal{E}}{R}e^{-t/\tau_L}~~~(\mathrm{turning~off}).\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-c0f2ac20a226c92f75b2193360ee77ec_l3.png)
Current falls to
 in the first time interval
in the first time interval 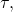 and to
and to 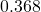 of the remainder toward zero in each subsequent time
of the remainder toward zero in each subsequent time 
11.5 Oscillations in an LC Circuit
- The energy transferred in an oscillatory manner between the capacitor and inductor in an
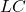 circuit occurs at an angular frequency
circuit occurs at an angular frequency 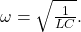
- The charge and current in the circuit are given by
![Rendered by QuickLaTeX.com \[q(t)=q_0\cos(\omega t+\phi),\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-af64683a865ffae3eaca73ef7cb39d02_l3.png)
![Rendered by QuickLaTeX.com \[i(t)=-\omega q_0\sin(\omega t+\phi).\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-a3ceb5c9be4b68bd18ba7d6d9d2f2f6e_l3.png)
11.6 RLC Series Circuits
- The underdamped solution for the capacitor charge in an
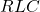 circuit is
circuit is
![Rendered by QuickLaTeX.com \[q(t)=q_0e^{-Rt/2L}\cos(\omega't+\phi).\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-2f107d6053672e7447290c9a2fdfd506_l3.png)
- The angular frequency given in the underdamped solution for the
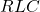 circuit is
circuit is
![Rendered by QuickLaTeX.com \[\omega'=\sqrt{\frac{1}{LC}-\left(\frac{R}{2L}\right)^2}.\]](https://openpress.usask.ca/app/uploads/quicklatex/quicklatex.com-4c053f9828fe95b8f3a88d6f984692d4_l3.png)
Answers to Check Your Understanding
11.1 ![]()
11.2 a. decreasing; b. increasing; Since the current flows in the opposite direction of the diagram, in order to get a positive emf on the left-hand side of diagram (a), we need to decrease the current to the left, which creates a reinforced emf where the positive end is on the left-hand side. To get a positive emf on the right-hand side of diagram (b), we need to increase the current to the left, which creates a reinforced emf where the positive end is on the right-hand side.
11.3 ![]()
11.4 a. ![]() ; b.
; b. ![]()
11.5 a. ![]() b.
b. ![]()
11.6 ![]()
11.8 a. ![]() ; b.
; b. ![]() ; c.
; c. ![]()
11.10 a. ![]() ; b.
; b. ![]() or
or ![]() ; c.
; c. ![]()
11.11 a. overdamped; b. ![]()
Conceptual Questions
11.1 Mutual Inductance
1. Show that ![]() and
and ![]() which are both expressions for self-inductance, have the same units.
which are both expressions for self-inductance, have the same units.
2. A ![]() inductor carries a current of
inductor carries a current of ![]() Describe how a
Describe how a ![]() emf can be induced across it.
emf can be induced across it.
3. The ignition circuit of an automobile is powered by a ![]() battery. How are we able to generate large voltages with this power source?
battery. How are we able to generate large voltages with this power source?
4. When the current through a large inductor is interrupted with a switch, an arc appears across the open terminals of the switch. Explain.
11.2 Self-Inductance and Inductors
5. Does self-inductance depend on the value of the magnetic flux? Does it depend on the current through the wire? Correlate your answers with the equation ![]()
6. Would the self-inductance of a ![]() long, tightly wound solenoid differ from the self-inductance per meter of an infinite, but otherwise identical, solenoid?
long, tightly wound solenoid differ from the self-inductance per meter of an infinite, but otherwise identical, solenoid?
7. Discuss how you might determine the self-inductance per unit length of a long, straight wire.
8. The self-inductance of a coil is zero if there is no current passing through the windings. True or false?
9. How does the self-inductance per unit length near the centre of a solenoid (away from the ends) compare with its value near the end of the solenoid?
11.3 Energy in a Magnetic Field
10. Show that ![]() has units of energy.
has units of energy.
11.4 RL Circuits
11. Use Lenz’s law to explain why the initial current in the ![]() circuit of Figure 11.4.1(b) is zero.
circuit of Figure 11.4.1(b) is zero.
12. When the current in the ![]() circuit of Figure 11.4.1(b) reaches its final value
circuit of Figure 11.4.1(b) reaches its final value ![]() what is the voltage across the inductor? Across the resistor?
what is the voltage across the inductor? Across the resistor?
13. Does the time required for the current in an ![]() circuit to reach any fraction of its steady-state value depend on the emf of the battery?
circuit to reach any fraction of its steady-state value depend on the emf of the battery?
14. An inductor is connected across the terminals of a battery. Does the current that eventually flows through the inductor depend on the internal resistance of the battery? Does the time required for the current to reach its final value depend on this resistance?
15. At what time is the voltage across the inductor of the ![]() circuit of Figure 14.12(b) a maximum?
circuit of Figure 14.12(b) a maximum?
16. In the simple ![]() circuit of Figure 11.4.1(b), can the emf induced across the inductor ever be greater than the emf of the battery used to produce the current?
circuit of Figure 11.4.1(b), can the emf induced across the inductor ever be greater than the emf of the battery used to produce the current?
17. If the emf of the battery of Figure 11.4.1(b) is reduced by a factor of ![]() by how much does the steady-state energy stored in the magnetic field of the inductor change?
by how much does the steady-state energy stored in the magnetic field of the inductor change?
18. A steady current flows through a circuit with a large inductive time constant. When a switch in the circuit is opened, a large spark occurs across the terminals of the switch. Explain.
19. Describe how the currents through ![]() and
and ![]() shown below vary with time after switch
shown below vary with time after switch ![]() is closed.
is closed.
20. Discuss possible practical applications of ![]() circuits.
circuits.
11.5 Oscillations in an LC Circuit
21. Do Kirchhoff’s rules apply to circuits that contain inductors and capacitors?
22. Can a circuit element have both capacitance and inductance?
23. In an ![]() circuit, what determines the frequency and the amplitude of the energy oscillations in either the inductor or capacitor?
circuit, what determines the frequency and the amplitude of the energy oscillations in either the inductor or capacitor?
11.6 RLC Series Circuits
24. When a wire is connected between the two ends of a solenoid, the resulting circuit can oscillate like an ![]() circuit. Describe what causes the capacitance in this circuit.
circuit. Describe what causes the capacitance in this circuit.
25. Describe what effect the resistance of the connecting wires has on an oscillating ![]() circuit.
circuit.
26. Suppose you wanted to design an ![]() circuit with a frequency of
circuit with a frequency of ![]() What problems might you encounter?
What problems might you encounter?
27. A radio receiver uses an ![]() circuit to pick out particular frequencies to listen to in your house or car without hearing other unwanted frequencies. How would someone design such a circuit?
circuit to pick out particular frequencies to listen to in your house or car without hearing other unwanted frequencies. How would someone design such a circuit?
Problems
11.1 Mutual Inductance
28. When the current in one coil changes at a rate of ![]() an emf of
an emf of ![]() is induced in a second, nearby coil. What is the mutual inductance of the two coils?
is induced in a second, nearby coil. What is the mutual inductance of the two coils?
29. An emf of ![]() is induced in a coil while the current in a nearby coil is decreasing at a rate of
is induced in a coil while the current in a nearby coil is decreasing at a rate of ![]() What is the mutual inductance of the two coils?
What is the mutual inductance of the two coils?
30. Two coils close to each other have a mutual inductance of ![]() If the current in one coil decays according to
If the current in one coil decays according to ![]() where
where ![]() and
and ![]() what is the emf induced in the second coil immediately after the current starts to decay? At
what is the emf induced in the second coil immediately after the current starts to decay? At ![]() ?
?
31. A coil of ![]() is wrapped around a long solenoid of cross-sectional area
is wrapped around a long solenoid of cross-sectional area ![]() The solenoid is
The solenoid is ![]() long and has
long and has ![]() (a) What is the mutual inductance of this system? (b) The outer coil is replaced by a coil of
(a) What is the mutual inductance of this system? (b) The outer coil is replaced by a coil of ![]() whose radius is three times that of the solenoid. What is the mutual inductance of this configuration?
whose radius is three times that of the solenoid. What is the mutual inductance of this configuration?
32. A ![]() solenoid is
solenoid is ![]() long and
long and ![]() in diameter. Inside the solenoid, a small
in diameter. Inside the solenoid, a small ![]() single-turn rectangular coil is fixed in place with its face perpendicular to the long axis of the solenoid. What is the mutual inductance of this system?
single-turn rectangular coil is fixed in place with its face perpendicular to the long axis of the solenoid. What is the mutual inductance of this system?
33. A toroidal coil has a mean radius of ![]() and a cross-sectional area of
and a cross-sectional area of ![]() ; it is wound uniformly with
; it is wound uniformly with ![]() A second toroidal coil of
A second toroidal coil of ![]() is wound uniformly over the first coil. Ignoring the variation of the magnetic field within a toroid, determine the mutual inductance of the two coils.
is wound uniformly over the first coil. Ignoring the variation of the magnetic field within a toroid, determine the mutual inductance of the two coils.
34. A solenoid of ![]() turns has length
turns has length ![]() and radius
and radius ![]() and a second smaller solenoid of
and a second smaller solenoid of ![]() turns has length
turns has length ![]() and radius
and radius ![]() The smaller solenoid is placed completely inside the larger solenoid so that their long axes coincide. What is the mutual inductance of the two solenoids?
The smaller solenoid is placed completely inside the larger solenoid so that their long axes coincide. What is the mutual inductance of the two solenoids?
11.2 Self-Inductance and Inductors
35. An emf of ![]() is induced across a coil when the current through it changes uniformly from
is induced across a coil when the current through it changes uniformly from ![]() to
to ![]() in
in ![]() What is the self-inductance of the coil?
What is the self-inductance of the coil?
36. The current shown in part (a) below is increasing, whereas that shown in part (b) is decreasing. In each case, determine which end of the inductor is at the higher potential.
37. What is the rate at which the current though a ![]() coil is changing if an emf of
coil is changing if an emf of ![]() is induced across the coil?
is induced across the coil?
38. When a camera uses a flash, a fully charged capacitor discharges through an inductor. In what time must the ![]() current through a
current through a ![]() inductor be switched on or off to induce a
inductor be switched on or off to induce a ![]() emf?
emf?
39. A coil with a self-inductance of ![]() carries a current that varies with time according to
carries a current that varies with time according to ![]() Find an expression for the emf induced in the coil.
Find an expression for the emf induced in the coil.
40. A solenoid ![]() long is wound with
long is wound with ![]() of wire. The cross-sectional area of the coil is
of wire. The cross-sectional area of the coil is ![]() What is the self-inductance of the solenoid?
What is the self-inductance of the solenoid?
41. A coil with a self-inductance of ![]() carries a current that decreases at a uniform rate
carries a current that decreases at a uniform rate ![]() What is the emf induced in the coil? Describe the polarity of the induced emf.
What is the emf induced in the coil? Describe the polarity of the induced emf.
42. The current ![]() through a
through a ![]() inductor varies with time, as shown below. The resistance of the inductor is
inductor varies with time, as shown below. The resistance of the inductor is ![]() Calculate the voltage across the inductor at
Calculate the voltage across the inductor at ![]()
![]() and
and ![]()
43. A long, cylindrical solenoid with ![]() has a radius of
has a radius of ![]() (a) Neglecting end effects, what is the self-inductance per unit length of the solenoid? (b) If the current through the solenoid changes at the rate
(a) Neglecting end effects, what is the self-inductance per unit length of the solenoid? (b) If the current through the solenoid changes at the rate ![]() what is the emf induced per unit length?
what is the emf induced per unit length?
44. Suppose that a rectangular toroid has ![]() windings and a self-inductance of
windings and a self-inductance of ![]() If
If ![]() what is the ratio of its outer radius to its inner radius?
what is the ratio of its outer radius to its inner radius?
45. What is the self-inductance per meter of a coaxial cable whose inner radius is ![]() and whose outer radius is
and whose outer radius is ![]() ?
?
11.3 Energy in a Magnetic Field
46. At the instant a current of ![]() is flowing through a coil of wire, the energy stored in its magnetic field is
is flowing through a coil of wire, the energy stored in its magnetic field is ![]() What is the self-inductance of the coil?
What is the self-inductance of the coil?
47. Suppose that a rectangular toroid has ![]() windings and a self-inductance of
windings and a self-inductance of ![]() If
If ![]() what is the current flowing through a rectangular toroid when the energy in its magnetic field is
what is the current flowing through a rectangular toroid when the energy in its magnetic field is ![]() ?
?
48. Solenoid ![]() is tightly wound while solenoid
is tightly wound while solenoid ![]() has windings that are evenly spaced with a gap equal to the diameter of the wire. The solenoids are otherwise identical. Determine the ratio of the energies stored per unit length of these solenoids when the same current flows through each.
has windings that are evenly spaced with a gap equal to the diameter of the wire. The solenoids are otherwise identical. Determine the ratio of the energies stored per unit length of these solenoids when the same current flows through each.
49. A ![]() inductor carries a current of
inductor carries a current of ![]() How much ice at
How much ice at ![]() could be melted by the energy stored in the magnetic field of the inductor? (Hint: Use the value
could be melted by the energy stored in the magnetic field of the inductor? (Hint: Use the value ![]() for ice.)
for ice.)
50. A coil with a self-inductance of ![]() and a resistance of
and a resistance of ![]() carries a steady current of
carries a steady current of ![]() (a) What is the energy stored in the magnetic field of the coil? (b) What is the energy per second dissipated in the resistance of the coil?
(a) What is the energy stored in the magnetic field of the coil? (b) What is the energy per second dissipated in the resistance of the coil?
51. A current of ![]() is flowing in a coaxial cable whose outer radius is five times its inner radius. What is the magnetic field energy stored in a
is flowing in a coaxial cable whose outer radius is five times its inner radius. What is the magnetic field energy stored in a ![]() length of the cable?
length of the cable?
11.4 RL Circuits
52. In Figure 11.4.1, ![]()
![]() and
and ![]() Determine (a) the time constant of the circuit, (b) the initial current through the resistor, (c) the final current through the resistor, (d) the current through the resistor when
Determine (a) the time constant of the circuit, (b) the initial current through the resistor, (c) the final current through the resistor, (d) the current through the resistor when ![]() and (e) the voltages across the inductor and the resistor when
and (e) the voltages across the inductor and the resistor when ![]()
53. For the circuit shown below, ![]()
![]() and
and ![]() After steady state is reached with
After steady state is reached with ![]() closed and
closed and ![]() open,
open, ![]() is closed and immediately thereafter (at
is closed and immediately thereafter (at ![]() )
) ![]() is opened. Determine (a) the current through
is opened. Determine (a) the current through ![]() at
at ![]() (b) the current through
(b) the current through ![]() at
at ![]() and (c) the voltages across
and (c) the voltages across ![]() and
and ![]() at
at ![]()
54. The current in the ![]() circuit shown here increases to
circuit shown here increases to ![]() of its steady-state value in
of its steady-state value in ![]() What is the time constant of the circuit?
What is the time constant of the circuit?
55. How long after switch ![]() is thrown does it take the current in the circuit shown to reach half its maximum value? Express your answer in terms of the time constant of the circuit.
is thrown does it take the current in the circuit shown to reach half its maximum value? Express your answer in terms of the time constant of the circuit.
56. Examine the circuit shown below in part (a). Determine ![]() at the instant after the switch is thrown in the circuit of (a), thereby producing the circuit of (b). Show that if
at the instant after the switch is thrown in the circuit of (a), thereby producing the circuit of (b). Show that if ![]() were to continue to increase at this initial rate, it would reach its maximum
were to continue to increase at this initial rate, it would reach its maximum ![]() in one time constant.
in one time constant.
57. The current in the ![]() circuit shown below reaches half its maximum value in
circuit shown below reaches half its maximum value in ![]() after the switch
after the switch ![]() is thrown. Determine (a) the time constant of the circuit and (b) the resistance of the circuit if
is thrown. Determine (a) the time constant of the circuit and (b) the resistance of the circuit if ![]()
58. Consider the circuit shown below. Find ![]()
![]() and
and ![]() when (a) the switch
when (a) the switch ![]() is first closed, (b) after the currents have reached steady-state values, and (c) at the instant the switch is reopened (after being closed for a long time).
is first closed, (b) after the currents have reached steady-state values, and (c) at the instant the switch is reopened (after being closed for a long time).
59. For the circuit shown below, ![]()
![]() and
and ![]() Find the values of
Find the values of ![]() and
and ![]() (a) immediately after switch
(a) immediately after switch ![]() is closed, (b) a long time after
is closed, (b) a long time after ![]() is closed, (c) immediately after
is closed, (c) immediately after ![]() is reopened, and (d) a long time after
is reopened, and (d) a long time after ![]() is reopened.
is reopened.
60. For the circuit shown below, find the current through the inductor ![]() after the switch is reopened.
after the switch is reopened.
61. Show that for the circuit shown below, the initial energy stored in the inductor, ![]() is equal to the total energy eventually dissipated in the resistor,
is equal to the total energy eventually dissipated in the resistor, ![]()
11.5 Oscillations in an LC Circuit
62. A ![]() capacitor is charged to
capacitor is charged to ![]() and then quickly connected to an
and then quickly connected to an ![]() inductor. Determine (a) the maximum energy stored in the magnetic field of the inductor, (b) the peak value of the current, and (c) the frequency of oscillation of the circuit.
inductor. Determine (a) the maximum energy stored in the magnetic field of the inductor, (b) the peak value of the current, and (c) the frequency of oscillation of the circuit.
63. The self-inductance and capacitance of an ![]() circuit are
circuit are ![]() and
and ![]() What is the angular frequency at which the circuit oscillates?
What is the angular frequency at which the circuit oscillates?
64. What is the self-inductance of an ![]() circuit that oscillates at
circuit that oscillates at ![]() when the capacitance is
when the capacitance is ![]() ?
?
65. In an oscillating ![]() circuit, the maximum charge on the capacitor is
circuit, the maximum charge on the capacitor is ![]() and the maximum current through the inductor is
and the maximum current through the inductor is ![]() (a) What is the period of the oscillations? (b) How much time elapses between an instant when the capacitor is uncharged and the next instant when it is fully charged?
(a) What is the period of the oscillations? (b) How much time elapses between an instant when the capacitor is uncharged and the next instant when it is fully charged?
66. The self-inductance and capacitance of an oscillating ![]() circuit are
circuit are ![]() and
and ![]() respectively. (a) What is the frequency of the oscillations? (b) If the maximum potential difference between the plates of the capacitor is
respectively. (a) What is the frequency of the oscillations? (b) If the maximum potential difference between the plates of the capacitor is ![]() what is the maximum current in the circuit?
what is the maximum current in the circuit?
67. In an oscillating ![]() circuit, the maximum charge on the capacitor is
circuit, the maximum charge on the capacitor is ![]() Determine the charge on the capacitor and the current through the inductor when energy is shared equally between the electric and magnetic fields. Express your answer in terms of
Determine the charge on the capacitor and the current through the inductor when energy is shared equally between the electric and magnetic fields. Express your answer in terms of ![]()
![]() and
and ![]()
68. In the circuit shown below, ![]() is opened and
is opened and ![]() is closed simultaneously. Determine (a) the frequency of the resulting oscillations, (b) the maximum charge on the capacitor, (c) the maximum current through the inductor, and (d) the electromagnetic energy of the oscillating circuit.
is closed simultaneously. Determine (a) the frequency of the resulting oscillations, (b) the maximum charge on the capacitor, (c) the maximum current through the inductor, and (d) the electromagnetic energy of the oscillating circuit.
69. An ![]() circuit in an AM tuner (in a car stereo) uses a coil with an inductance of
circuit in an AM tuner (in a car stereo) uses a coil with an inductance of ![]() and a variable capacitor. If the natural frequency of the circuit is to be adjustable over the range
and a variable capacitor. If the natural frequency of the circuit is to be adjustable over the range ![]() to
to ![]() (the AM broadcast band), what range of capacitance is required?
(the AM broadcast band), what range of capacitance is required?
11.6 RLC Series Circuits
70. In an oscillating ![]() circuit,
circuit, ![]()
![]() and
and ![]() What is the angular frequency of the oscillations?
What is the angular frequency of the oscillations?
71. In an oscillating ![]() circuit with
circuit with ![]()
![]() and
and ![]() how much time elapses before the amplitude of the oscillations drops to half its initial value?
how much time elapses before the amplitude of the oscillations drops to half its initial value?
72. What resistance ![]() must be connected in series with a
must be connected in series with a ![]() inductor of the resulting
inductor of the resulting ![]() oscillating circuit is to decay to
oscillating circuit is to decay to ![]() of its initial value of charge in
of its initial value of charge in ![]() cycles? To
cycles? To ![]() of its initial value in
of its initial value in ![]() cycles?
cycles?
Additional Problems
73. Show that the self-inductance per unit length of an infinite, straight, thin wire is infinite.
74. Two long, parallel wires carry equal currents in opposite directions. The radius of each wire is ![]() and the distance between the centres of the wires is
and the distance between the centres of the wires is ![]() Show that if the magnetic flux within the wires themselves can be ignored, the self-inductance of a length
Show that if the magnetic flux within the wires themselves can be ignored, the self-inductance of a length ![]() of such a pair of wires is
of such a pair of wires is
![]()
(Hint: Calculate the magnetic flux through a rectangle of length ![]() between the wires and then use
between the wires and then use ![]() )
)
75. A small, rectangular single loop of wire with dimensions ![]() and
and ![]() is placed, as shown below, in the plane of a much larger, rectangular single loop of wire. The two short sides of the larger loop are so far from the smaller loop that their magnetic fields over the smaller fields over the smaller loop can be ignored. What is the mutual inductance of the two loops?
is placed, as shown below, in the plane of a much larger, rectangular single loop of wire. The two short sides of the larger loop are so far from the smaller loop that their magnetic fields over the smaller fields over the smaller loop can be ignored. What is the mutual inductance of the two loops?
76. Suppose that a cylindrical solenoid is wrapped around a core of iron whose magnetic susceptibility is ![]() Using Equation 11.2.5, show that the self-inductance of the solenoid is given by
Using Equation 11.2.5, show that the self-inductance of the solenoid is given by ![]() where
where ![]() is its length,
is its length, ![]() its cross-sectional area, and
its cross-sectional area, and ![]() its total number of turns.
its total number of turns.
77. The solenoid of the preceding problem is wrapped around an iron core whose magnetic susceptibility is ![]() (a) If a current of
(a) If a current of ![]() flows through the solenoid, what is the magnetic field in the iron core? (b) What is the effective surface current formed by the aligned atomic current loops in the iron core? (c) What is the self-inductance of the filled solenoid?
flows through the solenoid, what is the magnetic field in the iron core? (b) What is the effective surface current formed by the aligned atomic current loops in the iron core? (c) What is the self-inductance of the filled solenoid?
78. A rectangular toroid with inner radius ![]() outer radius
outer radius ![]() height
height ![]() and
and ![]() is filled with an iron core of magnetic susceptibility
is filled with an iron core of magnetic susceptibility ![]() (a) What is the self-inductance of the toroid? (b) If the current through the toroid is
(a) What is the self-inductance of the toroid? (b) If the current through the toroid is ![]() what is the magnetic field at the centre of the core? (c) For this same
what is the magnetic field at the centre of the core? (c) For this same ![]() current, what is the effective surface current formed by the aligned atomic current loops in the iron core?
current, what is the effective surface current formed by the aligned atomic current loops in the iron core?
79. The switch ![]() of the circuit shown below is closed at
of the circuit shown below is closed at ![]() Determine (a) the initial current through the battery and (b) the steady-state current through the battery.
Determine (a) the initial current through the battery and (b) the steady-state current through the battery.
80. In an oscillating ![]() circuit,
circuit, ![]()
![]() and
and ![]() Initially, the capacitor has a charge of
Initially, the capacitor has a charge of ![]() and the current is zero. Calculate the charge on the capacitor (a) five cycles later and (b)
and the current is zero. Calculate the charge on the capacitor (a) five cycles later and (b) ![]() cycles later.
cycles later.
81. A ![]() inductor has
inductor has ![]() of current turned off in
of current turned off in ![]() (a) What voltage is induced to oppose this? (b) What is unreasonable about this result? (c) Which assumption or premise is responsible?
(a) What voltage is induced to oppose this? (b) What is unreasonable about this result? (c) Which assumption or premise is responsible?
Challenge Problems
82. A coaxial cable has an inner conductor of radius ![]() and outer thin cylindrical shell of radius
and outer thin cylindrical shell of radius ![]() A current
A current ![]() flows in the inner conductor and returns in the outer conductor. The self-inductance of the structure will depend on how the current in the inner cylinder tends to be distributed. Investigate the following two extreme cases. (a) Let current in the inner conductor be distributed only on the surface and find the self-inductance. (b) Let current in the inner cylinder be distributed uniformly over its cross-section and find the self-inductance. Compare with your results in (a).
flows in the inner conductor and returns in the outer conductor. The self-inductance of the structure will depend on how the current in the inner cylinder tends to be distributed. Investigate the following two extreme cases. (a) Let current in the inner conductor be distributed only on the surface and find the self-inductance. (b) Let current in the inner cylinder be distributed uniformly over its cross-section and find the self-inductance. Compare with your results in (a).
83. In a damped oscillating circuit the energy is dissipated in the resistor. The ![]() -factor is a measure of the persistence of the oscillator against the dissipative loss. (a) Prove that for a lightly damped circuit the energy,
-factor is a measure of the persistence of the oscillator against the dissipative loss. (a) Prove that for a lightly damped circuit the energy, ![]() in the circuit decreases according to the following equation.
in the circuit decreases according to the following equation.
![]()
where ![]()
(b) Using the definition of the ![]() -factor as energy divided by the loss over the next cycle, prove that
-factor as energy divided by the loss over the next cycle, prove that ![]() -factor of a lightly damped oscillator as defined in this problem is
-factor of a lightly damped oscillator as defined in this problem is
![]()
(Hint: For (b), to obtain ![]() divide
divide ![]() at the beginning of one cycle by the change
at the beginning of one cycle by the change ![]() over the next cycle.)
over the next cycle.)
84. The switch in the circuit shown below is closed at ![]() Find currents through (a)
Find currents through (a) ![]() (b)
(b) ![]() and (c) the battery as function of time.
and (c) the battery as function of time.
85. A square loop of side ![]() is placed
is placed ![]() from a long wire carrying a current that varies with time at a constant rate of
from a long wire carrying a current that varies with time at a constant rate of ![]() as shown below. (a) Use Ampère’s law and find the magnetic field as a function of time from the current in the wire. (b) Determine the magnetic flux through the loop. (c) If the loop has a resistance of
as shown below. (a) Use Ampère’s law and find the magnetic field as a function of time from the current in the wire. (b) Determine the magnetic flux through the loop. (c) If the loop has a resistance of ![]() how much induced current flows in the loop?
how much induced current flows in the loop?
86. A rectangular copper ring, of mass ![]() and resistance
and resistance ![]() is in a region of uniform magnetic field that is perpendicular to the area enclosed by the ring and horizontal to Earth’s surface. The ring is let go from rest when it is at the edge of the nonzero magnetic field region (see below). (a) Find its speed when the ring just exits the region of uniform magnetic field. (b) If it was let go at
is in a region of uniform magnetic field that is perpendicular to the area enclosed by the ring and horizontal to Earth’s surface. The ring is let go from rest when it is at the edge of the nonzero magnetic field region (see below). (a) Find its speed when the ring just exits the region of uniform magnetic field. (b) If it was let go at ![]() what is the time when it exits the region of magnetic field for the following values:
what is the time when it exits the region of magnetic field for the following values: ![]()
![]()
![]() and
and ![]() ? Assume the magnetic field of the induced current is negligible compared to
? Assume the magnetic field of the induced current is negligible compared to ![]()
Candela Citations
- Download for free at http://cnx.org/contents/7a0f9770-1c44-4acd-9920-1cd9a99f2a1e@8.1. Retrieved from: http://cnx.org/contents/7a0f9770-1c44-4acd-9920-1cd9a99f2a1e@8.1. License: CC BY: Attribution

