Chapter 10 Review

Key Terms

back emf
emf generated by a running motor, because it consists of a coil turning in a magnetic field; it opposes the voltage powering the motor

eddy current
current loop in a conductor caused by motional emf

electric generator
device for converting mechanical work into electric energy; it induces an emf by rotating a coil in a magnetic field

Faraday’s law
induced emf is created in a closed loop due to a change in magnetic flux through the loop

induced electric field
created based on the changing magnetic flux with time

induced emf
short-lived voltage generated by a conductor or coil moving in a magnetic field

Lenz’s law
direction of an induced emf opposes the change in magnetic flux that produced it; this is the negative sign in Far

day’s law
magnetic damping
drag produced by eddy currents

magnetic flux
measurement of the amount of magnetic field lines through a given area

motionally induced emf
voltage produced by the movement of a conducting wire in a magnetic field

peak emf
maximum emf produced by a generator

Key Equations

Magnetic flux \Phi_{\mathrm{m}}=\int_S\vec{\mathbf{B}}\cdot\hat{\mathbf{n}}dA
Faraday’s law \mathcal{E}=-N\frac{d\Phi_{\mathrm{m}}}{dt}
Motionally induced emf \mathcal{E}=Blv
Motional emf around a circuit \mathcal{E}=\oint\vec{\mathbf{E}}\cdot d\vec{\mathbf{l}}=-\frac{d\Phi_{\mathrm{m}}}{dt}
Emf produced by an electric generator \mathcal{E}=NBA\omega\sin(\omega t)

Summary

10.1 Faraday’s Law

  • The magnetic flux through an enclosed area is defined as the amount of field lines cutting through a surface area 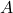A defined by the unit area vector.
  • The units for magnetic flux are webers, where 1~\mathrm{Wb}=1~\mathrm{T}\cdot\mathrm{m}^2.
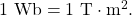
  • The induced emf in a closed loop due to a change in magnetic flux through the loop is known as Faraday’s law. If there is no change in magnetic flux, no induced emf is created.

10.2 Lenz’s Law

  • We can use Lenz’s law to determine the directions of induced magnetic fields, currents, and emfs.
  • The direction of an induced emf always opposes the change in magnetic flux that causes the emf, a result known as Lenz’s law.

10.3 Motional Emf

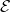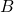  • The relationship between an induced emf \mathcal{E} in a wire moving at a constant speed v through a magnetic field B is given by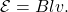 \mathcal{E}=Blv.
  • An induced emf from Faraday’s law is created from a motional emf that opposes the change in flux.

10.4 Induced Electric Fields

  • A changing magnetic flux induces an electric field.
  • Both the changing magnetic flux and the induced electric field are related to the induced emf from Faraday’s law.

10.5 Eddy Currents

  • Current loops induced in moving conductors are called eddy currents. They can create significant drag, called magnetic damping.
  • Manipulation of eddy currents has resulted in applications such as metal detectors, braking in trains or roller coasters, and induction cooktops.

10.6 Electric Generators and Back Emf

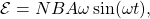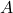  • An electric generator rotates a coil in a magnetic field, inducing an emf given as a function of time by \mathcal{E}=NBA\omega\sin(\omega t), where A is the area of an 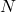N-turn coil rotated at a constant angular velocity \omega in a uniform magnetic field \vec{\mathbf{B}}.
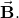
  • The peak emf of a generator is \mathcal{E}_0=NBA\omega.
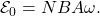
  • Any rotating coil produces an induced emf. In motors, this is called back emf because it opposes the emf input to the motor.

10.7 Applications of Electromagnetic Induction

  • Hard drives utilize magnetic induction to read/write information.
  • Other applications of magnetic induction can be found in graphics tablets, electric and hybrid vehicles, and in transcranial magnetic stimulation.

Answers to Check Your Understanding

10.1 1.1~\mathrm{T/s}

10.2 To the observer shown, the current flows clockwise as the magnet approaches, decreases to zero when the magnet is centered in the plane of the coil, and then flows counterclockwise as the magnet leaves the coil.

Figure shows a magnet that is moving into and through the loop with the South pole facing the loop. Position (a) corresponds to magnet approaching the loop; position (b) corresponds to the magnet directly into the loop. Position (c) corresponds to the magnet moving away from the loop. 

10.4 \mathcal{E}=Bl^2\omega/2, with O at a higher potential than S

10.5 1.5~\mathrm{V}

10.6 a. yes; b. Yes; however there is a lack of symmetry between the electric field and coil, making \oint\vec{\mathbf{E}}\cdot d\vec{\mathbf{l}} a more complicated relationship that can’t be simplified as shown in the example.

10.7 3.4\times10^{-3}~\mathrm{V/m}

10.8 P_1,~P_2,~P_4

10.9 a. 3.1\times10^{-6}~\mathrm{V}

Conceptual Questions

10.1 Faraday’s Law

1. A stationary coil is in a magnetic field that is changing with time. Does the emf induced in the coil depend on the actual values of the magnetic field?

2. In Faraday’s experiments, what would be the advantage of using coils with many turns?

3. A copper ring and a wooden ring of the same dimensions are placed in magnetic fields so that there is the same change in magnetic flux through them. Compare the induced electric fields and currents in the rings.

4. Discuss the factors determining the induced emf in a closed loop of wire.

5. (a) Does the induced emf in a circuit depend on the resistance of the circuit? (b) Does the induced current depend on the resistance of the circuit?

6. How would changing the radius of loop D shown below affect its emf, assuming C and D are much closer together compared to their radii?

 Figure shows a circuit that consists of a resistor, capacitor, opened switch and a loop C. A loop D is located next to the loop C.

7. Can there be an induced emf in a circuit at an instant when the magnetic flux through the circuit is zero?

8. Does the induced emf always act to decrease the magnetic flux through a circuit?

9. How would you position a flat loop of wire in a changing magnetic field so that there is no induced emf in the loop?

10. The normal to the plane of a single-turn conducting loop is directed at an angle \theta to a spatially uniform magnetic field \vec{\mathbf{B}}. It has a fixed area and orientation relative to the magnetic field. Show that the emf induced in the loop is given by \mathcal{E}=(dB/dt)(A\cos\theta), where A is the area of the loop.

10.2 Lenz’s Law

11. The circular conducting loops shown in the accompanying figure are parallel, perpendicular to the plane of the page, and coaxial. (a) When the switch S is closed, what is the direction of the current induced in D? (b) When the switch is opened, what is the direction of the current induced in loop D?

 Figure shows a circuit that consists of a resistor, capacitor, opened switch and a loop C. A loop D is located next to the loop C.

12. The north pole of a magnet is moved toward a copper loop, as shown below. If you are looking at the loop from above the magnet, will you say the induced current is circulating clockwise or counterclockwise?

Figure shows a magnet that is moving towards the loop with the North pole facing the loop.

13. The accompanying figure shows a conducting ring at various positions as it moves through a magnetic field. What is the sense of the induced emf for each of those positions?

 Figure shows the loop that moves through the area with the magnetic field perpendicular to it.

14. Show that \mathcal{E} and d\Phi_{\mathrm{m}}/dt have the same units.

15. State the direction of the induced current for each case shown below, observing from the side of the magnet.

Figure A shows a magnet that is moving towards the loop with the North pole facing the loop. Figure B shows a magnet that is moving away from the loop with the North pole facing the loop. Figure C shows a magnet that is moving towards the loop with the South pole facing the loop. Figure D shows a magnet that is hold constant with the North pole facing the loop. The loop is moving towards the magnet. Figure E shows a magnet that is hold constant with the North pole facing the loop. Loop is moving away from the magnet. Figure F shows a magnet that is hold constant with the North pole facing the loop. Loop is rotating clockwise.

10.3 Motional Emf

16. A bar magnet falls under the influence of gravity along the axis of a long copper tube. If air resistance is negligible, will there be a force to oppose the descent of the magnet? If so, will the magnet reach a terminal velocity?

17. Around the geographic North Pole (or magnetic South Pole), Earth’s magnetic field is almost vertical. If an airplane is flying northward in this region, which side of the wing is positively charged and which is negatively charged?

18. A wire loop moves translationally (no rotation) in a uniform magnetic field. Is there an emf induced in the loop?

10.4 Induced Electric Fields

19. Is the work required to accelerate a rod from rest to a speed v in a magnetic field greater than the final kinetic energy of the rod? Why?

20. The copper sheet shown below is partially in a magnetic field. When it is pulled to the right, a resisting force pulls it to the left. Explain. What happen if the sheet is pushed to the left?

 Figure shows a copper sheet pulled to the right through the uniform perpendicular magnetic field with the force F.

10.5 Eddy Currents

21. A conducting sheet lies in a plane perpendicular to a magnetic field \vec{\mathbf{B}} that is below the sheet. If \vec{\mathbf{B}} oscillates at a high frequency and the conductor is made of a material of low resistivity, the region above the sheet is effectively shielded from \vec{\mathbf{B}}. Explain why. Will the conductor shield this region from static magnetic fields?

22. Electromagnetic braking can be achieved by applying a strong magnetic field to a spinning metal disk attached to a shaft. (a) How can a magnetic field slow the spinning of a disk? (b) Would the brakes work if the disk was made of plastic instead of metal?

23. A coil is moved through a magnetic field as shown below. The field is uniform inside the rectangle and zero outside. What is the direction of the induced current and what is the direction of the magnetic force on the coil at each position shown?

Picture shows a coil is moved from the left to the right through a uniform magnetic field. Magnetic lines are perpendicular to the coil and are directed from the plane of the page.

Problems

10.1 Faraday’s Law

24. A 50-turn coil has a diameter of 15~\mathrm{cm}. The coil is placed in a spatially uniform magnetic field of magnitude 0.50~\mathrm{T} so that the face of the coil and the magnetic field are perpendicular. Find the magnitude of the emf induced in the coil if the magnetic field is reduced to zero uniformly in (a) 0.10~\mathrm{s}, (b) 1.0~\mathrm{s}, and (c) 60~\mathrm{s}.

25. Repeat your calculations of the preceding problem’s time of 0.1~\mathrm{s} with the plane of the coil making an angle of (a) 30^{\circ}, (b) 60^{\circ}, and (c) 90^{\circ} with the magnetic field.

26. A square loop whose sides are 6.0{\text -}\mathrm{cm} long is made with copper wire of radius 1.0~\mathrm{mm}. If a magnetic field perpendicular to the loop is changing at a rate of 5.0~\mathrm{mT/s}, what is the current in the loop?

27. The magnetic field through a circular loop of radius 10.0~\mathrm{cm} varies with time as shown below. The field is perpendicular to the loop. Plot the magnitude of the induced emf in the loop as a function of time.

Figure shows the magnetic field in milliTesla plotted as a function of time in ms. Magnetic field is zero when the time is equal to zero. It increases linearly with time reaching 3 milliTesla and 2 ms. It remains the same till 5 ms and then decreases linearly to 0 and 6 ms.

28. The accompanying figure shows a single-turn rectangular coil that has a resistance of 2.0~\Omega. The magnetic field at all points inside the coil varies according to B=B_0e^{-\alpha t} where B_0=0.25~\mathrm{T} and \alpha=200~\mathrm{Hz}. What is the current induced in the coil at (a) t=0.001~\mathrm{s}, (b) 0.002~\mathrm{s}, (c) 2.0~\mathrm{s}?

 Figure shows a square coil with the sides of 2.0 and 5.0 cm. A uniform magnetic field B is directed perpendicular to the coil.

29. How would the answers to the preceding problem change if the coil consisted of 20 closely spaced turns?

30. A long solenoid with n=10 turns per centimetre has a cross-sectional area of 5.0~\mathrm{cm^2} and carries a current of 0.25~\mathrm{A}. A coil with five turns encircles the solenoid. When the current through the solenoid is turned off, it decreases to zero in 0.050~\mathrm{s}. What is the average emf induced in the coil?

31. A rectangular wire loop with length a and width b lies in the xy-plane, as shown below. Within the loop there is a time-dependent magnetic field given by \vec{\mathbf{B}}(t)=C\left(x\cos\omega t\,\hat{\mathbf{i}}+y\sin\omega t\,\hat{\mathbf{k}}\right) with \vec{\mathbf{B}}(t) in tesla. Determine the emf induced in the loop as a function of time.

 Figure shows a rectangular wire loop with length a and width b lies in the xy-plane.

32. The magnetic field perpendicular to a single wire loop of diameter 10.0~\mathrm{cm} decreases from 0.50~\mathrm{T} to zero. The wire is made of copper and has a diameter of 2.0~\mathrm{mm} and length 1.0~\mathrm{cm}. How much charge moves through the wire while the field is changing?

10.2 Lenz’s Law

33. A single-turn circular loop of wire of radius 50~\mathrm{mm} lies in a plane perpendicular to a spatially uniform magnetic field. During a 0.10{\text -}\mathrm{s} time interval, the magnitude of the field increases uniformly from 200 to 300~\mathrm{mT}. (a) Determine the emf induced in the loop. (b) If the magnetic field is directed out of the page, what is the direction of the current induced in the loop?

34. When a magnetic field is first turned on, the flux through a 20-turn loop varies with time according to \Phi_{\mathrm{m}}=5.0t^2-2.0t, where \Phi_{\mathrm{m}} is in milliwebers, t is in seconds, and the loop is in the plane of the page with the unit normal pointing outward. (a) What is the emf induced in the loop as a function of time? What is the direction of the induced current at (b) t =0, (c) 0.10, (d) 1.0, and (e) 2.0~\mathrm{s}?

35. The magnetic flux through the loop shown in the accompanying figure varies with time according to \Phi_{\mathrm{m}}=2.00e^{-3t}\sin(120\pi t), where \Phi_{\mathrm{m}} is in milliwebers. What are the direction and magnitude of the current through the 5.00{\text -}\Omega resistor at (a) t=0, (b) t=2.17\times10^{-2}~\mathrm{s}, and (c) t=3.00~\mathrm{s}?

 Figure shows a loop with the magnetic flux perpendicular to the loop. Loop is connected to a 5 Ohm resistor.

36. Use Lenz’s law to determine the direction of induced current in each case.

Figure A shows a metal bar moving to the left in the perpendicular uniform magnetic field. Figure B shows a loop moving to the right in a parallel uniform magnetic field. Figure C shows a loop moved into a perpendicular uniform magnetic field. Figure D shows a metal bar moving to the right in the perpendicular uniform magnetic field. Figure E shows a loop located into an increasing perpendicular magnetic field. Figure F shows a loop located into a decreasing parallel magnetic field.

10.3 Motional Emf

37. An automobile with a radio antenna 1.0~\mathrm{m} long travels at 100.0~\mathrm{km/h} in a location where the Earth’s horizontal magnetic field is 5.5\times10^{-5}~\mathrm{T}. What is the maximum possible emf induced in the antenna due to this motion?

38. The rectangular loop of N turns shown below moves to the right with a constant velocity \vec{\mathbf{v}} while leaving the poles of a large electromagnet. (a) Assuming that the magnetic field is uniform between the pole faces and negligible elsewhere, determine the induced emf in the loop. (b) What is the source of work that produces this emf?

Figure shows the rectangular loop (short side has a length l, long side has a length a) of N turns that moves to the right with a constant velocity v while leaving the uniform magnetic field.

39. Suppose the magnetic field of the preceding problem oscillates with time according to B=B_0\sin\omega t. What then is the emf induced in the loop when its trailing side is a distance d from the right edge of the magnetic field region?

40. A coil of 1000 turns encloses an area of 25~\mathrm{cm}^2. It is rotated in 0.010~\mathrm{s} from a position where its plane is perpendicular to Earth’s magnetic field to one where its plane is parallel to the field. If the strength of the field is 6.0\times10^{-5}~\mathrm{T}, what is the average emf induced in the coil?

41. In the circuit shown in the accompanying figure, the rod slides along the conducting rails at a constant velocity \vec{\mathbf{v}}. The velocity is in the same plane as the rails and directed at an angle \theta to them. A uniform magnetic field \vec{\mathbf{B}} is directed out of the page. What is the emf induced in the rod?

 Figure shows the rod that slides along the conducting rails at a constant velocity v in a uniform perpendicular magnetic field. Distance between the rails is l. Angle between the direction of movement of the rod and the rails is theta.

42. The rod shown in the accompanying figure is moving through a uniform magnetic field of strength B=0.50~\mathrm{T} with a constant velocity of magnitude v=8.0~\mathrm{m/s}. What is the potential difference between the ends of the rod? Which end of the rod is at a higher potential?

 Figure shows the 5 cm long rod of that moves to the right at a constant velocity v in a uniform perpendicular magnetic field.

43. A 25{\text -}\mathrm{cm} rod moves at 5.0~\mathrm{m/s} in a plane perpendicular to a magnetic field of strength 0.25~\mathrm{T}. The rod, velocity vector, and magnetic field vector are mutually perpendicular, as indicated in the accompanying figure. Calculate (a) the magnetic force on an electron in the rod, (b) the electric field in the rod, and (c) the potential difference between the ends of the rod. (d) What is the speed of the rod if the potential difference is 1.0~\mathrm{V}?

 Figure shows the 25 cm long rod of that moves to the right at a constant velocity v in a uniform perpendicular magnetic field.

44. In the accompanying figure, the rails, connecting end piece, and rod all have a resistance per unit length of 2.0~\Omega/\mathrm{cm}. The rod moves to the left at v=3.0~\mathrm{m/s}. If B=0.75~\mathrm{T} everywhere in the region, what is the current in the circuit (a) when a=8.0~\mathrm{cm}? (b) when a=5.0~\mathrm{cm}? Specify also the sense of the current flow.

Figure shows the rod that slides to the left along the conducting rails at a constant velocity v in a uniform perpendicular magnetic field. Distance between the rails is 4 cm. The rod moves for the distance a.

45. The rod shown below moves to the right on essentially zero-resistance rails at a speed of v=3.0~\mathrm{m/s}. If B=0.75~\mathrm{T} everywhere in the region, what is the current through the 5.0{\text -}\Omega resistor? Does the current circulate clockwise or counterclockwise?

 Figure shows the rod that slides to the right along the conducting rails at a constant velocity v in a uniform perpendicular magnetic field. Distance between the rails is 4 cm. The rails are connected through the 5 Ohm resistor.

46. Shown below is a conducting rod that slides along metal rails. The apparatus is in a uniform magnetic field of strength 0.25~\mathrm{T}, which is directly into the page. The rod is pulled to the right at a constant speed of 5.0~\mathrm{m/s} by a force \vec{\mathbf{F}}. The only significant resistance in the circuit comes from the 2.0{\text -}\Omega resistor shown. (a) What is the emf induced in the circuit? (b) What is the induced current? Does it circulate clockwise or counter clockwise? (c) What is the magnitude of \vec{\mathbf{F}}? (d) What are the power output of \vec{\mathbf{F}} and the power dissipated in the resistor?

 Figure shows the rod that is pulled to the right along the conducting rails by the force F in a uniform perpendicular magnetic field. Distance between the rails is 4 cm. The rails are connected through the 2 Ohm resistor.

13.4 Induced Electric Fields

47. Calculate the induced electric field in a 50-turn coil with a diameter of 15~\mathrm{cm} that is placed in a spatially uniform magnetic field of magnitude 0.50~\mathrm{T} so that the face of the coil and the magnetic field are perpendicular. This magnetic field is reduced to zero in 0.10 seconds. Assume that the magnetic field is cylindrically symmetric with respect to the central axis of the coil.

48. The magnetic field through a circular loop of radius 10.0~\mathrm{cm} varies with time as shown in the accompanying figure. The field is perpendicular to the loop. Assuming cylindrical symmetry with respect to the central axis of the loop, plot the induced electric field in the loop as a function of time.

49. The current I through a long solenoid with n turns per meter and radius R is changing with time as given by dI/dt. Calculate the induced electric field as a function of distance r from the central axis of the solenoid.

50. Calculate the electric field induced both inside and outside the solenoid of the preceding problem if I=I_0\sin\omega t.

51. Over a region of radius R, there is a spatially uniform magnetic field \vec{\mathbf{B}}. (See below.) At t=0, B=1.0~\mathrm{T}, after which it decreases at a constant rate to zero in 30~\mathrm{s}. (a) What is the electric field in the regions where r\leq R and r\geq R during that 30{\text -}\mathrm{s} interval? (b) Assume that R=10.0~\mathrm{cm}. How much work is done by the electric field on a proton that is carried once clock wise around a circular path of radius 5.0~\mathrm{cm}? (c) How much work is done by the electric field on a proton that is carried once counterclockwise around a circular path of any radius r\geqR? (d) At the instant when B=0.50~\mathrm{T}, a proton enters the magnetic field at A, moving a velocity \vec{\mathbf{v}} (v=5.0\times10^6~\mathrm{m/s}), as shown. What are the electric and magnetic forces on the proton at that instant?

 Figure shows a proton carried into a uniform magnetic field with a radius R.

52. The magnetic field at all points within the cylindrical region whose cross-section is indicated in the accompanying figure starts at 1.0~\mathrm{T} and decreases uniformly to zero in 20~\mathrm{s}. What is the electric field (both magnitude and direction) as a function of r, the distance from the geometric centre of the region?

 Figure shows a uniform magnetic field with a radius of 20 centimeters.

53. The current in a long solenoid of radius 3~\mathrm{cm} is varied with time at a rate of 2~\mathrm{A/s}. A circular loop of wire of radius 5~\mathrm{cm} and resistance 2~\Omega surrounds the solenoid. Find the electrical current induced in the loop.

54. The current in a long solenoid of radius 3~\mathrm{cm} and 20~\mathrm{turns/cm} is varied with time at a rate of 2~\mathrm{A/s}. Find the electric field at a distance of 4~\mathrm{cm} from the centre of the solenoid.

10.6 Electric Generators and Back Emf

55. Design a current loop that, when rotated in a uniform magnetic field of strength 0.10~\mathrm{T}, will produce an emf \mathcal{E}=\mathcal{E}_0\sin\omega t, where \mathcal{E}_0=110~\mathrm{V} and \omega=120\pi~\mathrm{rad/s}.

56. A flat, square coil of 20~\mathrm{turns} that has sides of length 15.0~\mathrm{cm} is rotating in a magnetic field of strength 0.050~\mathrm{T}. If the maximum emf produced in the coil is 30.0~\mathrm{mV}, what is the angular velocity of the coil?

57. A 50{\text -}\mathrm{turn} rectangular coil with dimensions 0.15~\mathrm{m}\times0.40~\mathrm{m} rotates in a uniform magnetic field of magnitude 0.75~\mathrm{T} at 3600~\mathrm{rev/min}. (a) Determine the emf induced in the coil as a function of time. (b) If the coil is connected to a 1000{\text -}\Omega resistor, what is the power as a function of time required to keep the coil turning at 3600~\mathrm{rpm}? (c) Answer part (b) if the coil is connected to a 2000{\text -}\Omega resistor.

58. The square armature coil of an alternating current generator has 200~\mathrm{turns} and is 20.0~\mathrm{cm} on side. When it rotates at 3600~\mathrm{rpm}, its peak output voltage is 120~\mathrm{V}. (a) What is the frequency of the output voltage? (b) What is the strength of the magnetic field in which the coil is turning?

59. A flip coil is a relatively simple device used to measure a magnetic field. It consists of a circular coil of N~\mathrm{turns} wound with fine conducting wire. The coil is attached to a ballistic galvanometer, a device that measures the total charge that passes through it. The coil is placed in a magnetic field \vec{\mathbf{B}} such that its face is perpendicular to the field. It is then flipped through 180^{\circ}, and the total charge Q that flows through the galvanometer is measured. (a) If the total resistance of the coil and galvanometer is R, what is the relationship between B and Q? Because the coil is very small, you can assume that \vec{\mathbf{B}} is uniform over it. (b) How can you determine whether or not the magnetic field is perpendicular to the face of the coil?

60. The flip coil of the preceding problem has a radius of 3.0~\mathrm{cm} and is wound with 40~\mathrm{turns} of copper wire. The total resistance of the coil and ballistic galvanometer is 0.20~\Omega. When the coil is flipped through 180^{\circ} in a magnetic field \vec{\mathbf{B}}, a change of 0.090~\mathrm{C} flows through the ballistic galvanometer. (a) Assuming that \vec{\mathbf{B}} and the face of the coil are initially perpendicular, what is the magnetic field? (b) If the coil is flipped through 90^{\circ}, what is the reading of the galvanometer?

61. A 120{\text -}\mathrm{V}, series-wound motor has a field resistance of 80~\Omega and an armature resistance of 10~\Omega. When it is operating at full speed, a back emf of 75~\mathrm{V} is generated. (a) What is the initial current drawn by the motor? When the motor is operating at full speed, where are (b) the current drawn by the motor, (c) the power output of the source, (d) the power output of the motor, and (e) the power dissipated in the two resistances?

62. A small series-wound dc motor is operated from a 12{\text -}\mathrm{V} car battery. Under a normal load, the motor draws 4.0~\mathrm{A}, and when the armature is clamped so that it cannot turn, the motor draws 24~\mathrm{A}. What is the back emf when the motor is operating normally?

Additional Problems

63. Shown in the following figure is a long, straight wire and a single-turn rectangular loop, both of which lie in the plane of the page. The wire is parallel to the long sides of the loop and is 0.50~\mathrm{m} away from the closer side. At an instant when the emf induced in the loop is 2.0~\mathrm{V}, what is the time rate of change of the current in the wire?

Figure shows a long, straight wire and a single-turn rectangular wire loop with length 3.0 m and width 0.5cm, both of which lie in the plane of the page. The wire is parallel to the long sides of the loop and is 0.50 m away from the closer side.

64. A metal bar of mass 500~\mathrm{g} slides outward at a constant speed of 1.5~\mathrm{cm/s} over two parallel rails separated by a distance of 30~\mathrm{cm} which are part of a U-shaped conductor. There is a uniform magnetic field of magnitude 2~\mathrm{T} pointing out of the page over the entire area. The railings and metal bar have an equivalent resistance of 150~\Omega. (a) Determine the induced current, both magnitude and direction. (b) Find the direction of the induced current if the magnetic field is pointing into the page. (c) Find the direction of the induced current if the magnetic field is pointed into the page and the bar moves inwards.

65. A current is induced in a circular loop of radius 1.5~\mathrm{cm} between two poles of a horseshoe electromagnet when the current in the electromagnet is varied. The magnetic field in the area of the loop is perpendicular to the area and has a uniform magnitude. If the rate of change of magnetic field is 10~\mathrm{T/s}, find the magnitude and direction of the induced current if resistance of the loop is 25~\Omega.

66. A metal bar of length 25~\mathrm{cm} is placed perpendicular to a uniform magnetic field of strength 3~\mathrm{T}. (a) Determine the induced emf between the ends of the rod when it is not moving. (b) Determine the emf when the rod is moving perpendicular to its length and magnetic field with a speed of 50~\mathrm{cm/s}.

67. A coil with 50~\mathrm{turns} and area 10~\mathrm{cm}^2 is oriented with its plane perpendicular to a 0.75{\text -}\mathrm{T} magnetic field. If the coil is flipped over (rotated through 180^{\circ}) in 0.20~\mathrm{s}, what is the average emf induced in it?

68. A 2{\text -}\mathrm{turn} planer loop of flexible wire is placed inside a long solenoid of n turns per meter that carries a constant current I_0. The area A of the loop is changed by pulling on its sides while ensuring that the plane of the loop always remains perpendicular to the axis of the solenoid. If n=500~\mathrm{turns/m}, I_0=20~\mathrm{A}, and A=20~\mathrm{cm}^2, what is the emf induced in the loop when dA/dt=100?

69. The conducting rod shown in the accompanying figure moves along parallel metal rails that are 25{\text -}\mathrm{cm} apart. The system is in a uniform magnetic field of strength 0.75~\mathrm{T}, which is directed into the page. The resistances of the rod and the rails are negligible, but the section PQ has a resistance of 0.25~\Omega. (a) What is the emf (including its sense) induced in the rod when it is moving to the right with a speed of 5.0~\mathrm{m/s}? (b) What force is required to keep the rod moving at this speed? (c) What is the rate at which work is done by this force? (d) What is the power dissipated in the resistor?

Figure shows the rod that slides to the right along the conducting rails at a constant velocity 5 meters per second in a uniform perpendicular magnetic field. Distance between the rails is 25 cm. The rails are connected trough the 0.25 Ohm resistor.

70. A circular loop of wire of radius 10~\mathrm{cm} is mounted on a vertical shaft and rotated at a frequency of 5 cycles per second in a region of uniform magnetic field of 2 Gauss perpendicular to the axis of rotation. (a) Find an expression for the time-dependent flux through the ring. (b) Determine the time-dependent current through the ring if it has a resistance of 10~\Omega.

Figure shows a circular loop of wire mounted on a vertical shaft and rotated in a region of uniform magnetic field perpendicular to the axis of rotation.

71. The magnetic field between the poles of a horseshoe electromagnet is uniform and has a cylindrical symmetry about an axis from the middle of the South Pole to the middle of the North Pole. The magnitude of the magnetic field changes as a rate of dB/dt due to the changing current through the electromagnet. Determine the electric field at a distance r from the centre.

72. A long solenoid of radius a with n turns per unit length is carrying a time-dependent current I(t)=\sin\omega t, where I_0 and \omega are constants. The solenoid is surrounded by a wire of resistance R that has two circular loops of radius b with b>a (see the following figure). Find the magnitude and direction of current induced in the outer loops at time t=0.

 Figure shows a long solenoid of radius a which is surrounded by a wire of resistance R that has two circular loops of larger radius b.

73. A 120{\text -}\mathrm{V}, series-wound dc motor draws 0.50~\mathrm{A} from its power source when operating at full speed, and it draws 2.0~\mathrm{A} when it starts. The resistance of the armature coils is 10~\Omega. (a) What is the resistance of the field coils? (b) What is the back emf of the motor when it is running at full speed? (c) The motor operates at a different speed and draws 1.0~\mathrm{A} from the source. What is the back emf in this case?

74. The armature and field coils of a series-wound motor have a total resistance of 3.0~\Omega. When connected to a 120{\text -}\mathrm{V} source and running at normal speed, the motor draws 4.0~\mathrm{A}. (a) How large is the back emf? (b) What current will the motor draw just after it is turned on? Can you suggest a way to avoid this large initial current?

Challenge Problems

75. A copper wire of length L is fashioned into a circular coil with N turns. When the magnetic field through the coil changes with time, for what value of N is the induced emf a maximum?

76. A 0.50{\text -}\mathrm{kg} copper sheet drops through a uniform horizontal magnetic field of 1.5~\mathrm{T}, and it reaches a terminal velocity of 2.0~\mathrm{m/s}. (a) What is the net magnetic force on the sheet after it reaches terminal velocity? (b) Describe the mechanism responsible for this force. (c) How much power is dissipated as Joule heating while the sheet moves at terminal velocity?

77. A circular copper disk of radius 7.5~\mathrm{cm} rotates at 2400~\mathrm{rpm} around the axis through its centre and perpendicular to its face. The disk is in a uniform magnetic field \vec{\mathbf{B}} of strength 1.2~\mathrm{T} that is directed along the axis. What is the potential difference between the rim and the axis of the disk?

78. A short rod of length a moves with its velocity \vec{\mathbf{v}} parallel to an infinite wire carrying a current I (see below). If the end of the rod nearer the wire is a distance b from the wire, what is the emf induced in the rod?

 Figure shows a short rod of length a that moves with its velocity v parallel to an infinite wire carrying a current I. Rod is moving at a distance b from the wire.

79. A rectangular circuit containing a resistance R is pulled at a constant velocity \vec{\mathbf{v}} away from a long, straight wire carrying a current I_0 (see below). Derive an equation that gives the current induced in the circuit as a function of the distance x between the near side of the circuit and the wire.

 Figure shows a rectangular circuit containing a resistor R that is pulled at a constant velocity v away from a long, straight wire carrying a current I0. Circuit is currently located at a distance x from the wire. Long side of the circuit is of the length a. It is parallel to the wire and contains the resistor. Short side of the circuit is of the length b.

80. Two infinite solenoids cross the plane of the circuit as shown below. The radii of the solenoids are 0.10 and 0.20~\mathrm{m}, respectively, and the current in each solenoid is changing such that dB/dt=50.0~\mathrm{T/s}. What are the currents in the resistors of the circuit?

 Figure shows two infinite solenoids that cross the plane of the circuit. The circuit consists of three resistors: 8 Ohm resistor at the center and two 4 Ohm resistors at the edges.

81. An eight-turn coil is tightly wrapped around the outside of the long solenoid as shown below. The radius of the solenoid is 2.0~\mathrm{cm} and it has 10~\mathrm{turns/cm}. The current through the solenoid increases according to I=I_0(1-e^{-\alpha t}), where I_0=4.0~\mathrm{A} and \alpha=2.0\times10^{-2}~\mathrm{s}^{-1}. What is the emf induced in the coil when (a) t=0, (b) t=1.0\times10^2~\mathrm{s}, and (c) t\rightarrow\infty?

Figure shows a coil that is tightly wrapped around the outside of the long solenoid.

82. Shown below is a long rectangular loop of width w, length l, mass m, and resistance R. The loop starts from rest at the edge of a uniform magnetic field \vec{\mathbf{B}}. and is pushed into the field by a constant force \vec{\mathbf{F}}. Calculate the speed of the loop as a function of time.

 Figure shows a long rectangular loop of width w. The loop starts from rest at the edge of a uniform magnetic field and is pushed into the field by a constant force F.

83. A square bar of mass m and resistance R is sliding without friction down very long, parallel conducting rails of negligible resistance (see below). The two rails are a distance l apart and are connected to each other at the bottom of the incline by a zero-resistance wire. The rails are inclined at an angle \theta, and there is a uniform vertical magnetic field \vec{\mathbf{B}} throughout the region. (a) Show that the bar acquires a terminal velocity given by v=\frac{mgR\sin\theta}{B^2l^2\cos^2\theta}. (b) Calculate the work per unit time done by the force of gravity. (c) Compare this with the power dissipated in the Joule heating of the bar. (d) What would happen if \vec{\mathbf{B}} were reversed?

Figure shows a square sliding down very long, parallel conducting rails. The two rails are a distance l apart and are inclined at an angle theta. There is a uniform vertical magnetic field B throughout the region.

84. The accompanying figure shows a metal disk of inner radius r_1 and other radius r_2 rotating at an angular velocity \vec{\omega} while in a uniform magnetic field directed parallel to the rotational axis. The brush leads of a voltmeter are connected to the dark’s inner and outer surfaces as shown. What is the reading of the voltmeter?

Figure shows a metal disk rotating at an angular velocity in a uniform magnetic field directed parallel to the rotational axis. The brush leads of a voltmeter are connected to the disk’s inner and outer surfaces.

85. A long solenoid with 10~\mathrm{turns/cm} is placed inside a copper ring such that both objects have the same central axis. The radius of the ring is 10.0~\mathrm{cm}, and the radius of the solenoid is 5.0~\mathrm{cm}. (a) What is the emf induced in the ring when the currentI through the solenoid is 5.0~\mathrm{A} and changing at a rate of 100~\mathrm{A/s}? (b) What is the emf induced in the ring when I=2.0~\mathrm{A} and dI/dt=100~\mathrm{A/s}? (c) What is the electric field inside the ring for these two cases? (d) Suppose the ring is moved so that its central axis and the central axis of the solenoid are still parallel but no longer coincide. (You should assume that the solenoid is still inside the ring.) Now what is the emf induced in the ring? (e) Can you calculate the electric field in the ring as you did in part (c)?

86. The current in the long, straight wire shown in the accompanying figure is given by I=I_0\sin\omega t, where I_o=15~\mathrm{A} and \omega=120\pi~\mathrm{rad/s}. What is the current induced in the rectangular loop at (a) t=0 and (b) t=2.1\times10^{-3}~\mathrm{s}? The resistance of the loop is 2.0~\Omega.

 Figure shows a rectangular circuit located next to a long, straight wire carrying a current I. Circuit is located at a distance 5 cm from the wire. Side of the circuit that is 8 cm long is parallel to the wire, side of the circuit that is 5 cm long is perpendicular to the wire.

87. A 500{\text -}\mathrm{turn} coil with a 0.250{\text -}\mathrm{m}^2 area is spun in Earth’s 5.00\times10^{-5}~\mathrm{T} magnetic field, producing a 12.0{\text -}\mathrm{kV} maximum emf. (a) At what angular velocity must the coil be spun? (b) What is unreasonable about this result? (c) Which assumption or premise is responsible?

88. A circular loop of wire of radius 10~\mathrm{cm} is mounted on a vertical shaft and rotated at a frequency of 5 cycles per second in a region of uniform magnetic field of 2\times10^{-4}~\mathrm{T} perpendicular to the axis of rotation. (a) Find an expression for the time-dependent flux through the ring (b) Determine the time-dependent current through the ring if it has a resistance of 10~\Omega.

89. A long solenoid of radius aa with nn turns per unit length is carrying a time-dependent current I(t)=I_0\sin\omega t where I_0 and \omega are constants. The solenoid is surrounded by a wire of resistance R that has two circular loops of radius b with b>a. Find the magnitude and direction of current induced in the outer loops at time t=0.

90. A rectangular copper loop of mass 100~\mathrm{g} and resistance 0.20~\Omega is in a region of uniform magnetic field that is perpendicular to the area enclosed by the ring and horizontal to Earth’s surface (see below). The loop is let go from rest when it is at the edge of the nonzero magnetic field region. (a) Find an expression for the speed when the loop just exits the region of uniform magnetic field. (b) If it was let go at t=0, what is the time when it exits the region of magnetic field for the following values: a=25~\mathrm{cm}, b=50~\mathrm{cm}, B=3~\mathrm{T}, g=9.8~\mathrm{m/s}^2? Assume that the magnetic field of the induced current is negligible compared to 3~\mathrm{T}.

 Figure A shows rectangular loop with the sides a and b in a region of uniform magnetic field that is perpendicular to the area enclosed by the loop and horizontal to Earth’s surface. Figure B shows rectangular loop that due to the force of gravity left a region of uniform magnetic field.

91. A metal bar of mass m slides without friction over two rails a distance D apart in the region that has a uniform magnetic field of magnitude B_0 and direction perpendicular to the rails (see below). The two rails are connected at one end to a resistor whose resistance is much larger than the resistance of the rails and the bar. The bar is given an initial speed of v_0. It is found to slow down. How far does the bar go before coming to rest? Assume that the magnetic field of the induced current is negligible compared to B_0.

 Figure shows metal bar that slides over two rails a distance D apart in the region that has a uniform magnetic field of magnitude in direction perpendicular to the rails. The two rails are connected at one end to a resistor R.

92. A time-dependent uniform magnetic field of magnitude B(t) is confined in a cylindrical region of radius R. A conducting rod of length 2D is placed in the region, as shown below. Show that the emf between the ends of the rod is given by \frac{dB}{dt}D\sqrt{R^2-D^2}. (Hint: To find the emf between the ends, we need to integrate the electric field from one end to the other. To find the electric field, use Faraday’s law as “Ampère’s law for E.”)

License

Icon for the Creative Commons Attribution 4.0 International License

Introduction to Electricity, Magnetism, and Circuits Copyright © 2018 by Daryl Janzen is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.