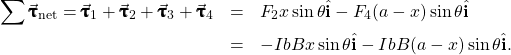8.5 Force and Torque on a Current Loop
LEARNING OBJECTIVES
- Evaluate the net force on a current loop in an external magnetic field
- Evaluate the net torque on a current loop in an external magnetic field
- Define the magnetic dipole moment of a current loop
Motors are the most common application of magnetic force on current-carrying wires. Motors contain loops of wire in a magnetic field. When current is passed through the loops, the magnetic field exerts torque on the loops, which rotates a shaft. Electrical energy is converted into mechanical work in the process. Once the loop’s surface area is aligned with the magnetic field, the direction of current is reversed, so there is a continual torque on the loop (Figure 8.5.2). This reversal of the current is done with commutators and brushes. The commutator is set to reverse the current flow at set points to keep continual motion in the motor. A basic commutator has three contact areas to avoid and dead spots where the loop would have zero instantaneous torque at that point. The brushes press against the commutator, creating electrical contact between parts of the commutator during the spinning motion.
(Figure 8.5.1) ![]()
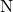 and
and 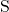 ) are opposite in direction as determined by the right-hand rule-1. Therefore, the loop has a net torque and rotates to the position shown in (b). (b) The brushes now touch the commutator segments so that no current flows through the loop. No torque acts on the loop, but the loop continues to spin from the initial velocity given to it in part (a). By the time the loop flips over, current flows through the wires again but now in the opposite direction, and the process repeats as in part (a). This causes continual rotation of the loop.
) are opposite in direction as determined by the right-hand rule-1. Therefore, the loop has a net torque and rotates to the position shown in (b). (b) The brushes now touch the commutator segments so that no current flows through the loop. No torque acts on the loop, but the loop continues to spin from the initial velocity given to it in part (a). By the time the loop flips over, current flows through the wires again but now in the opposite direction, and the process repeats as in part (a). This causes continual rotation of the loop.In a uniform magnetic field, a current-carrying loop of wire, such as a loop in a motor, experiences both forces and torques on the loop. Figure 8.5.2 shows a rectangular loop of wire that carries a current ![]() and has sides of lengths
and has sides of lengths ![]() and
and ![]() . The loop is in a uniform magnetic field:
. The loop is in a uniform magnetic field: ![]() . The magnetic force on a straight current-carrying wire of length
. The magnetic force on a straight current-carrying wire of length ![]() is given by
is given by ![]() . To find the net force on the loop, we have to apply this equation to each of the four sides. The force on side
. To find the net force on the loop, we have to apply this equation to each of the four sides. The force on side ![]() is
is
where the direction has been determined with the RHR-1. The current in side ![]() flows in the opposite direction to that of side
flows in the opposite direction to that of side ![]() , so
, so
The currents in sides ![]() and
and ![]() are perpendicular to
are perpendicular to ![]() and the forces on these sides are
and the forces on these sides are
We can now find the net force on the loop:
Although this result ![]() has been obtained for a rectangular loop, it is far more general and holds for current-carrying loops of arbitrary shapes; that is, there is no net force on a current loop in a uniform magnetic field.
has been obtained for a rectangular loop, it is far more general and holds for current-carrying loops of arbitrary shapes; that is, there is no net force on a current loop in a uniform magnetic field.
(Figure 8.5.2) ![]()
To find the net torque on the current loop shown in Figure 8.5.2, we first consider ![]() and
and ![]() . Since they have the same line of action and are equal and opposite, the sum of their torques about any axis is zero (see Fixed-Axis Rotation). Thus, if there is any torque on the loop, it must be furnished by
. Since they have the same line of action and are equal and opposite, the sum of their torques about any axis is zero (see Fixed-Axis Rotation). Thus, if there is any torque on the loop, it must be furnished by ![]() and
and ![]() . Let’s calculate the torques around the axis that passes through point
. Let’s calculate the torques around the axis that passes through point ![]() of Figure 8.5.2 (a side view of the coil) and is perpendicular to the plane of the page. The point
of Figure 8.5.2 (a side view of the coil) and is perpendicular to the plane of the page. The point ![]() is a distance
is a distance ![]() from side
from side ![]() and a distance
and a distance ![]() from side
from side ![]() of the loop. The moment arms of
of the loop. The moment arms of ![]() and
and ![]() are
are ![]() and
and ![]() , respectively, so the net torque on the loop is
, respectively, so the net torque on the loop is
This simplifies to
where ![]() is the area of the loop.
is the area of the loop.
Notice that this torque is independent of ![]() ; it is therefore independent of where point
; it is therefore independent of where point ![]() is located in the plane of the current loop. Consequently, the loop experiences the same torque from the magnetic field about any axis in the plane of the loop and parallel to the
is located in the plane of the current loop. Consequently, the loop experiences the same torque from the magnetic field about any axis in the plane of the loop and parallel to the ![]() -axis.
-axis.
A closed-current loop is commonly referred to as a magnetic dipole and the term ![]() is known as its magnetic dipole moment
is known as its magnetic dipole moment ![]() . Actually, the magnetic dipole moment is a vector that is defined as
. Actually, the magnetic dipole moment is a vector that is defined as
where ![]() is a unit vector directed perpendicular to the plane of the loop (see Figure 8.5.2). The direction of
is a unit vector directed perpendicular to the plane of the loop (see Figure 8.5.2). The direction of ![]() is obtained with the RHR-2—if you curl the fingers of your right hand in the direction of current flow in the loop, then your thumb points along
is obtained with the RHR-2—if you curl the fingers of your right hand in the direction of current flow in the loop, then your thumb points along ![]() . If the loop contains
. If the loop contains ![]() turns of wire, then its magnetic dipole moment is given by
turns of wire, then its magnetic dipole moment is given by
In terms of the magnetic dipole moment, the torque on a current loop due to a uniform magnetic field can be written simply as
This equation holds for a current loop in a two-dimensional plane of arbitrary shape.
Using a calculation analogous to that found in Capacitance for an electric dipole, the potential energy of a magnetic dipole is
EXAMPLE 8.5.1
Forces and Torques on Current-Carrying Loops
A circular current loop of radius ![]() carries a current of
carries a current of ![]() . (a) What is the magnitude of its magnetic dipole moment? (b) If the dipole is oriented at
. (a) What is the magnitude of its magnetic dipole moment? (b) If the dipole is oriented at ![]() degrees to a uniform magnetic field of magnitude
degrees to a uniform magnetic field of magnitude ![]() , what is the magnitude of the torque it experiences and what is its potential energy?
, what is the magnitude of the torque it experiences and what is its potential energy?
Strategy
The dipole moment is defined by the current times the area of the loop. The area of the loop can be calculated from the area of the circle. The torque on the loop and potential energy are calculated from identifying the magnetic moment, magnetic field, and angle oriented in the field.
Solution
a. The magnetic moment ![]() is calculated by the current times the area of the loop or
is calculated by the current times the area of the loop or ![]() .
.
![]()
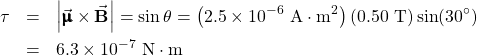
![]()
Significance
The concept of magnetic moment at the atomic level is discussed in the next chapter. The concept of aligning the magnetic moment with the magnetic field is the functionality of devices like magnetic motors, whereby switching the external magnetic field results in a constant spinning of the loop as it tries to align with the field to minimize its potential energy.
CHECK YOUR UNDERSTANDING 8.4
In what orientation would a magnetic dipole have to be to produce (a) a maximum torque in a magnetic field? (b) A maximum energy of the dipole?
Candela Citations
- Download for free at http://cnx.org/contents/7a0f9770-1c44-4acd-9920-1cd9a99f2a1e@8.1. Retrieved from: http://cnx.org/contents/7a0f9770-1c44-4acd-9920-1cd9a99f2a1e@8.1. License: CC BY: Attribution