1.5 Cost Allocation Methods
Once you’ve applied a costing procedure to determine the costs of a project, the next step in making a good budget is to allocate those costs into appropriate categories. This can be done in many ways, but two common approaches are function-based costing and activity-based costing. This section will introduce you to using both of these approaches.
1.5.1 Function-Based Costing
Function-based costing works by using production drivers (e.g. machine hours, labour hours) to assign costs to various departments (e.g. sales, marketing, manufacturing). This is the method that businesses have traditionally used to track their costs, since it provides a straightforward breakdown of where money is spent within the company, and it provides good information about a business’s function for external investors. We can think of function-based costing as a three step process:
- Identify Unit-Level Drivers
Function-based costing is highly dependent on the concept of units and unit costs, where the unit is the basic quantity of the good or service being produced. For a shoe factory the unit would be a pair of shoes, and for a tour company a unit might be a single 1-hour helicopter tour. The cost drivers used in this costing method are exclusively “unit-level drivers”; that is, they are variable costs, highly correlated to the number of units produced. All overhead costs which are variables costs (e.g. utilities) are assigned using driver tracing . Any overhead costs which are fixed costs, or are not correlated to unit production (e.g. building lease, equipment depreciation), must be allocated on a different basis .
In order to determine accurate unit costs , the first step must be identifying the unit-level drivers of those costs. As examples, these drivers could include machine-hours, labour hours, or simply the number of units produced.
- Estimate Driver Capacity
Once you have identified the unit-level drivers for each department , you must estimate its capacity, or number of units that you expect to be produced over a certain period . You might find it useful to consider various types of estimates, as illustrated here with the example of a gas station :
Expected Capacity: On any given day, the gas station expects to sell about 12,000 litres of gasoline, based on an average from the last full year of sales. The expected capacity is a short term estimate of capacity.
Normal Capacity: If gas prices are unusually low this year, the gas station might expect gas consumption to dip again if prices rise back to their typical levels. Therefore, they might expect to sell about 11,000 litres of gasoline per day over the next 5 years. The normal capacity is a longer term estimate of capacity.
Theoretical Capacity: Suppose the city was hit with a hurricane warning, and every car in the city rushed to the station to fuel up so that they could leave town. From opening to close, every pump in the gas station would be occupied, and they could sell every last drop of their 50,000 litre their fuel reservoir in a single day. The theoretical capacity is an absolute maximum for capacity.
Practical Capacity: If the station operated with high efficiency, so that even during peak hours of the day there were always open pumps and no customers were turned away to the competing gas station across the road, they could sell 14,000 litres of gas per day. The practical capacity is an estimate of the maximum capacity under efficient operation.
- Estimate Overhead Cost
The final step in function-based costing is to estimate overhead costs. First, the total overhead costs for the operation must be determined. Then, these overhead costs can be used to determine a department-wide overhead rate based on a unit level driver. Finally, these overhead costs can be assigned to individual products based on the amount of the unit level driver used per product .
Let’s try an example to see how function-based costing can be applied:
Cost Allocation Example
Not-A-Real-Mountain Manufacturing produces two models of downhill skis: the Faceplant and the ShowOff. The production of the skis is a two-step process, beginning in the Production Department and finishing in the Assembly Department. The table below lists the direct labour hours (DLH), machine hours (M/C Hours), total direct costs, and overhead costs incurred during manufacturing 10,000 sets of skis. After identifying the most relevant cost drivers, the company decides that overhead should be applied to the production process on the basis of machine hours, and to the assembly process on the basis of direct labour hours. Using the data given:
- a) determine an appropriate overhead rate per set of skis, for both models, and
- b) find the total amount that should be charged for an order of 700 Faceplants and 250 ShowOffs, including direct costs and overhead.
| Production | Assembly | |||||
| Faceplant | Show-off | Total | Faceplant | Show-off | Total | |
| No. of units | 8000 | 2000 | 10000 | 8000 | 2000 | 10000 |
| Direct Labour hrs | 450 | 190 | 640 | 2850 | 1250 | 4100 |
| M/C hours | 1300 | 420 | 1720 | 350 | 150 | 500 |
| Direct Cost | $192,000 | $94,000 | $286,000 | $104,000 | $68,000 | $172,000 |
| Overhead | $65,000 | $24,000 | ||||
Part a)
Step 1: Begin by finding plant-wide overhead rates for production and assembly, by dividing our total overhead costs by the total machine hours and total direct labour hours, respectively.
![]()
![]()
Step 2: Assign these numbers based on the number of machine hours or labour hours expended on each unit to find production and assembly overhead rates for an individual set of each model of skis.
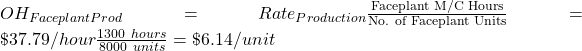
![]()
![]()
![]()
Step 3: Find total overhead rates by combining the production and assembly rates for each model.
![]()
![]()
The overhead rate for the Faceplant model is $8.22/unit, and for the ShowOff model is $11.60/unit.
Part b)
Step 1: Find the direct cost per unit for each model, combining production and assembly costs.
![]()
![]()
Step 2: Find the price for the order in part b) using the direct cost and overhead cost per unit.
![]()
![]()
Total Cost = $54,804
The total amount that should be charged for this order of skis is $54,804 plus a markup for profit.
1.5.2 Activity-Based Costing
Activity-based costing uses resource drivers (e.g. time, space) and activity drivers (e.g. units produced) to assign costs to various activities. Here we define an activity as some specific action or set of related actions that perform work for a project, such as shipping or receiving. Since this method breaks down costs by the specific activities of the company, rather than by department, which it makes it a good approach for internal decision making because it shows the resources used and income generated by the individual activities of the business. This approach is newer than function-based costing, and can also be more time consuming to carry out, because it may require costs from several departments to be estimated and recombined. We can think of activity-based costing as a four step process :
- Identify Activities
There may be many possible ways to differentiate and categorize the activities completed by a certain department. To ensure that you make useful distinctions, it is usually helpful to interview managers or representatives of the department in question, who will be most familiar with the work done in their area. With this information, you can develop an “activity dictionary” which tracks the specifics of each activity , including its attributes, metrics (drivers), and who it is used by. Depending on the complexity of a business and the level of detail in the analysis, you may choose to record hundreds of activities.
- Assign Costs to Activities
Once you have arrived at a list of activities, the next step is determine a cost for each one based on the resources which it consumes. As with function-based costing, these costs can either be directly apparent, or determined with the use of cost drivers if costs are split between several activities. This will create an “activity rate” which represents the cost of performing the activity.
- Assign Activity Costs to Other Activities
This is an intermediate step, wherein any activities whose costs are dependent on other activities are assigned those costs in turn. For example, if one activity is “advertising” and another is “sales and marketing”, the cost of advertising might be added as one part of the sales and marketing costs.
- Assign Costs to Products
Finally, the cost of a product can be found by summing the activity costs that contribute to its production. These activity costs are found by multiplying activity rates by the practical capacity (the amount an activity is used) of their activity, and determining the amount of each activity used by a given product.
Let’s took a look at a question similar to our previous example, this time using activity based costing.
Activity Based Costing Example
Totally-A-Real-Mountain Manufacturing produces two models of downhill skis: the SafeSlope and the Widowmaker, which are manufactured in the same department. The table below lists the direct labour hours (DLH), machine hours (M/C Hours), total direct costs, and overhead costs (which are broken down into four activities: machining, maintenance, assembly, and testing) for a production run of 10,000 sets of skis. After identifying the most relevant activity drivers, the company decides that machining and maintenance are a function of machine hours, assembly is a function of direct labour hours, and testing is a function of the number of units produced. Using the data given:
a) determine an appropriate overhead rate per set of skis, for both models, and
b) find the total amount that should be charged for an order of 600 SafeSlopes and 150 Widowmakers, including direct costs and overhead.
| SafeSlope | Widowmaker | Total | |
| No. of units | 5500 | 4500 | 10000 |
| Direct labour hours | 300 | 250 | 550 |
| M/C Hours | 1100 | 800 | 1900 |
| Direct Cost | $165,000 | $139,500 | $304,500 |
| Machining | $12,000 | $11,500 | $23,500 |
| Maintenance | $4,000 | $3,500 | $7,500 |
| Assembly | $10,000 | $1,500 | $11,500 |
| Testing | $20,000 | $500 | $20,500 |
Part a)
Step 1: Find the proportion of costs for each of the four overhead-generating activities to the costs of their activity drivers.
![]()
![]()
![]()
![]()
Step 2: Using the data from the 10,000 unit run, find direct labour hours, machine hours, and direct cost per unit for each model of skis.
![]()
![]()
![]()
![]()
![]()
![]()
Step 3: Combine the rates calculated above to find unit overhead costs for the two models.
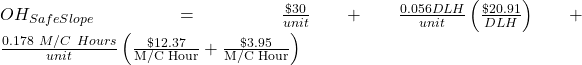
![]()
The overhead rates for the SafeSlope and the Widowmaker should be $10.92/unit and $9.87/unit, respectively.
Part b)
Step 1: Find the price for the order in part b) by combining direct and overhead costs to get a total unit cost for each model, and then multiplying by the number of units in the order.
![]()
![]()
![]()
![]()
The total price charged for the order should be $30,682.50 plus a markup for profit.
Gut Check: Is this a good answer? We followed all of the steps in the right order, so we should expect this amount to cover the total expenses of producing these skis. But look at the assumptions that Totally-A-Real-Mountain made about their activities: do the costs for machining, maintenance, assembly, and testing really correlate with the activity drivers they were assigned? You should notice that the assembly and testing costs for the Widowmaker are far lower than for the SafeSlope, even though their labour costs, direct costs, and units produced were extremely similar. If the costs of these activities were truly related, this should not be the case.
Since we are using one general rate for assembly and testing costs, we will be overestimating the overhead cost for Widowmakers, which use these activities far more, and underestimating the overhead cost for SafeSlopes, which use these activities far more. The order in part b) requests four times more SafeSlopes than Widowmakers, so our underestimation of these overhead costs may mean that Totally-A-Real-Mountain is not charging enough, and actually losing money on this order.
The bottom line is that choosing cost drivers and activity drivers to allocate overhead should be done carefully, because associating costs with unrelated drivers can lead to inaccurate estimates and loss of capital.

