15
Joel Bruneau and Clinton Mahoney
Learning Objectives
- Explain differentiated pricing and describe the three types of price discrimination
- Describe first-degree price discrimination and the challenges that make it hard
- Describe third-degree price discrimination and its effect on profits
- Describe second-degree price discrimination and how it overcomes the identification problem
- Define two-part tariffs and tie-in sales and how they work as price discrimination mechanisms
- Define bundling, versioning and hurdles and how each works to increase firm profits
Module 15: Pricing Strategies
Policy Example: Should Public Universities Charge Everyone the Same Price?
Modern public universities, like their private counterparts, publish a tuition price each year that few students actually pay. Most students receive internal grants and awards that reduce their costs well below the list price. The amount of this reduction in price varies dramatically and is closely related to the income of the student’s family.
{where is the graph “Here’s What Students Actually Pay for College”}
Unlike their private counterparts, public universities in the United States receive public funding and are expected, in return, to provide affordable college education for residents of the states they serve. Are their pricing policies antithetical to their mission? Are they fair? Do they support the mission of the institution? These are questions we will seek to answer by studying the practice of price discrimination.
Exploring the Policy Question
- Does charging tuition based on family income support or undermine the mission of public universities
- Is charging tuition based on family income fair?
15.1 Market Power and Price Discrimination
Learning Objective 15.1: Explain differentiated pricing and describe the three types of price discrimination.
15.2 Perfect or First-Degree Price Discrimination
Learning Objective 15.2: Describe first-degree price discrimination and the challenges that make it hard.
15.3 Group Price Discrimination or Third-Degree Price Discrimination
Learning Objective 15.3: Describe third-degree price discrimination and its effect on profits.
15.4 Quantity Discounts or Second-Degree Price Discrimination
Learning Objective 15.4: Describe second-degree price discrimination and how it overcomes the identification problem.
15.5 Two-Part Tariffs and Tie-In Sales
Learning Objective 15.5: Define two-part tariffs and tie-in sales and how they work as price discrimination mechanisms.
15.6 Bundling, Versioning and Hurdles
Learning Objective 15.6: Define bundling, versioning and hurdles and how each works to increase firm profits.
15.1 Market Power and Price Discrimination
Learning Objective 15.1: Explain differentiated pricing and describe the three types of price discrimination.
Firms that have market power face demand curves that are downward sloping. We call such firms price makers, since the shape of the demand curve gives them choices about the prices they charge. Monopolists of the type examined in Module 14, are simple monopolists: monopolists that are limited to a single price at which all of the output they produce is sold. This limitation leads simple monopolists to limit output so that they can maintain a higher price for all of their goods or services. This limitation in output creates deadweight loss, the lost surplus from transactions that don’t happen but that for which positive total surplus is possible. What we will see in this module is that firms with market power that are able to differentiate their consumers based on their demands or willingness-to-pay for the goods and services may be able to charge different prices for their goods and services. This practice is called differentiated pricing: selling the same good or service for different prices to different consumers. Differentiated pricing can come in many forms from a car dealership that negotiates prices with consumers selling the same model car for different prices to different customers, to a movie theater that offers a student price and an adult price, to volume discounts where consumer who buy multiple units qualify for lower per-unit prices such as a sale on socks that are either $4 a pair or $10 for three pairs. Differentiated pricing can also come in the form of bundling, selling a set of goods for a single price, and product differentiation, selling different versions of a product for different prices that do not reflect production cost differences.
These more sophisticated pricing strategies are the topic of this module and economists call the practice of charging different prices for the same good to different consumers price discrimination. Price discrimination often leads to higher profits for firms and to higher output as the incentive to constrain output to maintain a higher price for all units is no longer there. Price discrimination allows discriminating firms to capture more or all of the consumer surplus and the deadweight loss that results from a single price, and is therefore a strategy that increases profits. As we will see in this module, price discrimination can be hard because it requires firms to know information about consumers’ demands, and to be able to prevent resale of goods by a consumer who was charged a low price to a consumer who is being charged a high price.
Price discrimination is characterized by three main categories in economics. Perfect price discrimination, or first-degree price discrimination, is a type of pricing strategy that charges every consumer a price equal to his or her willingness-to-pay. Firms that can do this can extract the entire consumer surplus and all of the deadweight loss for themselves and can extract all potential profit from a market. This type of price discrimination is rare because it requires that firms can deduce each consumer’s willingness-to-pay and change then a price equal to it. Though it might be a hypothetical extreme, many firms try and change customers a price that is based on willingness-to-pay even if they can’t reach their exact willingness to pay. Car dealers that set final prices through negotiations or haggling are an example of this.
When firms are unable to ascertain individual willingness-to-pay but know something about the average demands among different distinguishable groups, they can practice group price discrimination, or third-degree price discrimination: charging different prices for the same good or service to different groups or different types of people. Movie theater pricing is a good example of this type of price discrimination where they often have different prices for kids, students, adults and seniors. This type of price discrimination requires only that firms are able to ascertain group membership and prevent resale from one group to the other.
When firms are unable to determine the willingness-to-pay of individuals or categorize individuals into groups based on average demand they can often employ pricing strategies that get consumers to self-select different prices based on their demands through the use of quantity discounts. Quantity discounts or second-degree price discrimination is when firms charge a lower price per unit to consumers who purchase larger amounts of the good.
It is important to understand that any firm with pricing power can potentially price discriminate but we focus in this module on monopolists to focus on how these pricing strategies are potentially profit enhancing. It is also important to note that not all price differentials are evidence of price discrimination. If price differentials simply reflect the actual cost differential. For example, a store might offer a single pair of socks for $4 or three pairs for $10 which could be clever price discrimination, however a mail-order retailer might make the same offer knowing that it costs $1 to prepare the shipment regardless of how many pairs of socks are ordered. So the socks are being sold for $3 plus the preparation charge and the price difference is simply a reflection of this fixed cost divided by the number of pairs of socks.
15.2 Perfect or First-Degree Price Discrimination
Learning Objective 15.2: Describe first-degree price discrimination and the challenges that make it hard.
Imagine a firm with market power that can prevent the resale of its goods and is able to ascertain each of its customers’ reservation price or maximum willingness-to-pay. If they are able to sell their goods at individual prices, the very best they can do in each transaction is to charge each customer exactly their reservation price. This ensures maximum revenue from each transaction as well as ensuring that the entire available surplus is captured by the firm as producer surplus.
Consider the simple example of a firm makes designer, gold plated, smart-phones that has only five potential customers, each of whom would purchase exactly one unit if the price is at or below their reservation price. The table below lists the customers and their reservation prices
|
Consumer |
Reservation Price |
|
1 |
$10,000 |
|
2 |
$8,000 |
|
3 |
$6,000 |
|
4 |
$4,000 |
|
5 |
$2,000 |
In the case of a simple monopolist the firm does not know the reservation prices of their consumers, in this case the firm does know then reservation prices of individual consumers. Suppose in addition that the firm has a marginal cost of producing a unit of a good of $3,000. The graph that illustrates this table is given in Figure 15.2.1. In this figure we see that at a price of $10,000, the firm would sell exactly one unit because there is only one consumer who has a reservation price that high.
Figure 15.2.1: First-Degree Price Discrimination
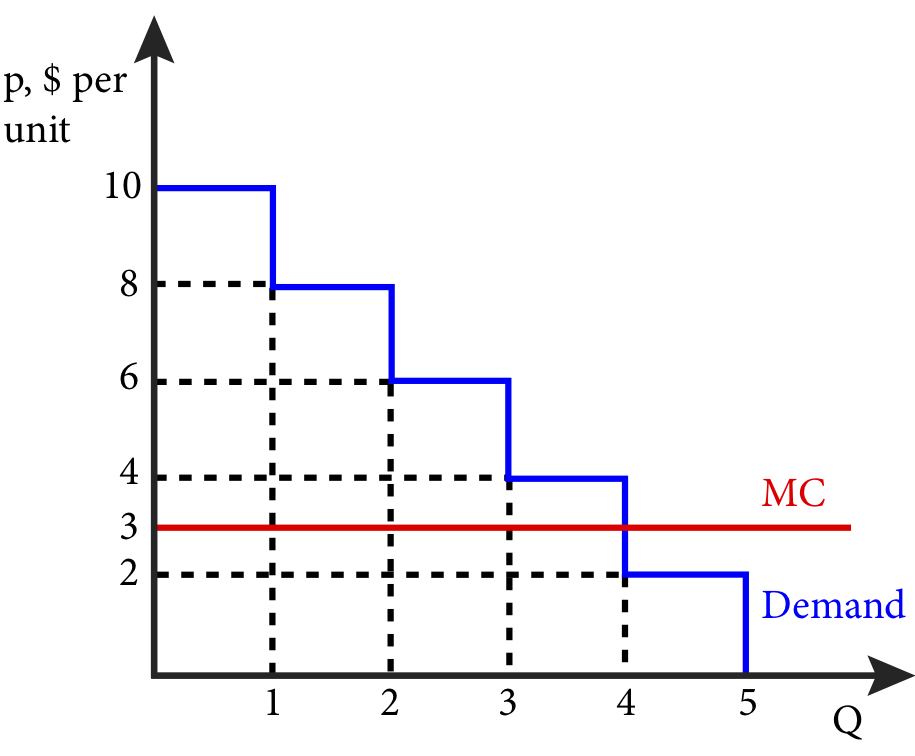
If the firm can practice perfect or first-degree price discrimination it means that they know each consumers reservation price and can prevent resale so they firm can charge consumer one $10, consumer two $8, consumer three $6, consumer four $4, and even consumer five $2. However, since consumer fives reservation price is below the marginal cost of production the firm will choose not to sell to consumer five. The marginal revenue is $10 for the first unit, $8 for the second unit, $6 for the third unit and $4 for the fourth unit. The surplus created by the sale of the first unit is the difference between the reservation price and the marginal cost: $10 – $3 = $7. This surplus is captured entirely by the firm making it all producer surplus. The producer surplus from the sale of the second good is $5, $3 from the sale of the third good and $1 from the sale of the fourth. Total producer surplus is the sum of these and equals $16, which is the area above the MC curve and below the demand curve. Notice that the marginal revenue curve is the same as the demand curve, which means this perfect price discriminating monopolist is producing at the point where marginal revenue equals marginal cost, four units.
Interestingly, the amount of output the firm produced is equal to the amount a perfectly competitive firm would produce and there is no deadweight loss. All the surplus that is possible to create in this market is created. The difference is, of course, that the firm captures the entire surplus for itself. So consumer surplus actually falls relative to a simple monopolist but total surplus and producer surplus increases.
Calculus Appendix
To understand the firm’s optimal output decision we can start with the knowledge that a perfect price discriminator charges each customer their reservation price or p=D(Q) where D(Q) is the inverse demand curve and Q is the firms output. Since the firm is charging each customer their reservation price their total revenue is the area under the demand curve up to the point of total output or:
[latex]\color{green}TR(Q)=\int ^Q_0D(z)dz[/latex]
Profit is total revenue minus the total cost of producing Q units of output, so the firms objective function is to maximize profit by choosing Q:
[latex]\color{green}\frac{max\,\pi (Q)}{Q}=\int ^Q_0D(z)dz[/latex]
The first-order condition that characterizes a maximum is:
So the firm will choose the Q where the demand curve and the marginal cost curve intersect, which is the same as a perfectly competitive firm.
This is an ideal situation for a firm with market power, but does it actually happen in the real world? It is rare to see it in its purest, most perfect form but some examples come close. Bargaining over price is one example. Sellers might not be able to tell exact willingness to pay but can become skilled in making educated guesses. Consider the case of new car sales. When a customer walks into an auto showroom and is greeted by a salesperson, that salesperson is already making inferences about the customer’s reservation price. Casual conversation about what the customer lives, works, their family are all potential clues. The haggling itself is another signal as low reservation price individuals will likely haggle quite strenuously and high reservation price individuals might not haggle much at all. In the end, each customer walks out of the dealership paying a different price where that price difference is related to reservation price.
Another good example of first-degree price discrimination is higher education. Colleges and universities are some of the best price discriminators around. It is estimated that less than a third of all U.S. college students pays full tuition. Most students routinely fill out a form to apply for student financial aid that reveals a lot about their family’s financial situation and therefore their reservation price or ability to pay. Through the use of grants, scholarships and subsidized loans each student is given a price of attending a college or university that is tailored to them.
15.3 Group Price Discrimination or Third-Degree Price Discrimination
Learning Objective 15.3: Describe third-degree price discrimination and its effect on profits.
In general, most firms with market power are unable to determine individual consumers’ reservation prices and charge them individual prices. However, firms might know something about the average reservation prices of identifiable groups. For example, it is generally true that, on average, retired individuals have less income than prime working age adults and are likely to have lower reservation prices across a number of goods like admission to movie theaters. If purchases are in person, group membership can be determined through identification such as a driver’s license. This solves the identification problem but the arbitrage problem remains. To maintain price differentials firms must be able prevent resale from members of the low price group to members of the high priced group. Often such resale is prevented naturally by transaction costs, the economic costs of buying and selling a good or service beyond the price itself. For example in the car dealership example, it would take time and effort to find a buyer for a car that was just purchased, there would be bureaucratic costs associated with the switching the registration of the car and in many states sales tax would have to be paid for both transactions. Since this is a cumbersome and costly process, there is unlikely to be much arbitrage in the new car market and differential pricing will be able to be sustained. In the case of movie theaters however, it is not hard to imagine a group of enterprising kids who purchase youth tickets and then wait outside the theater and sell them to adults at a premium over the youth price but at a discount over the adult price. This is why most movie theaters have ticket sellers and ticket takers who inspect tickets as patrons enter the theater to be sure adults have adult tickets.
To understand how group price discrimination works, consider the following example of book prices in the United States versus the United Kingdom. The book Steve Jobs was released in 2011 in both the U.S. and the U.K. In the U.S. the cover price of the book was $30, in the U.K. the cover price was £25, which at the time equaled $40. The reason for the price differential was likely due to the demand for the book in the U.S. being quite different than the demand in the U.K. To see this suppose that the marginal cost of production was $5 in both countries and that the demand for the book in the U.K. was QUK=75-pUK, and the demand for the book in the U.S. was QUS= 110-2pUS, where quantities are expressed in the thousands. Publishing companies are monopolists in the publication of a specific copyrighted book and therefore have market power in the market for that book. They are also quite sophisticated and we can safely assume they estimated the demand in both markets. They see that demand differs and since the two markets are geographically distinct, it makes it possible to chance different prices to the two groups by charging different prices in the two markets. Arbitrage is possible but the transactions costs associated with buying books in one market and shipping them to the other means that any attempts to arbitrage will probably be insignificant.
Calculus Appendix
To understand the multimarket monopolists optimal output decisions in each market consider the monopolist’s optimization problem. If the firm’s costs are common across markets, then we can write the total cost function as a function of the sum of the output for both markets, TC(Q1+Q2). Thus the profit maximization problem looks like this:
[latex]\color{green}\frac{maxπ(Q1,Q2)}{Q1,Q2}=TR_1(Q_1)+TR_2(Q_2)-TC(Q_1+Q_2)[/latex]
The first-order conditions that characterize the optimal solution are:
[latex]\color{green}\frac{\partial \pi }{\partial Q1}=\frac{dTR_1}{dQ1}-\frac{dTRC}{dQ}\frac{\partial Q}{\partial Q1}=0[/latex]
[latex]\color{green}\frac{\partial \pi }{\partial Q_2}=\frac{dTR_2}{dQ_2}-\frac{dTRC}{dQ}\frac{\partial Q}{\partial Q_2}=0[/latex]
Rearranging terms and noticing that the first term is the marginal revenue and the second term is marginal cost, we get:
MR1=MC
MR2=MC
Or the simple monopolists solution in both markets.
In each individual market, or to each group, the firm acts as a simple monopolist: charging a single price to all consumers in the market or group. So to figure out the profit maximizing price and quantity for the two markets, we simply have to solve the monopolist’s profit maximization problem. We start by solving for the inverse demand functions:
|
U.K. Market |
U.S. Market |
|
QUK=75-pUK |
QUS=110-2pUS
|
|
pUK=75-QUK |
2pUS=110-QUS
|
|
|
pUS=55-1/2 QUS
|
Because these are both linear demand curves, we know that the marginal revenue curves have the same vertical intercept as the inverse demand curves but have twice the slope. Thus the marginal revenue functions are the following:
|
U.K. Market |
U.S. Market |
|
pUK=75-QUK |
pUS=55-1/2 QUS
|
|
MRUK=75-2QUK |
MRUS=55-QUS
|
To find the profit maximizing price and quantity in each market we have to apply the profit maximization rule which says that the profit maximizing output level is reached when MR=MC:
|
U.K. Market |
U.S. Market |
|
MRUK=MCUK |
MRUS=MCUS
|
|
75-2QUK=5 |
55-QUS=5
|
|
70=2QUK |
50=QUS |
|
QUK=35 |
QUS=50 |
To find the price we simply plug these quantities into the inverse demand functions:
|
U.K. Market |
U.S. Market |
|
pUK=75-QUK |
pUS=55-1/2 QUS
|
|
pUK=75-35 |
pUS=55-1/2 50 |
|
pUK=$40 |
pUS=55-25 |
|
|
pUS=$30 |
The profit maximizing price for the publisher is $40 in the U.K market and $30 in the U.S. market.
Graphically the solution is shown in the Figure 15.3.1.
Figure 15.3.1: Group or Third-Degree Price Discrimination in Book Publishing
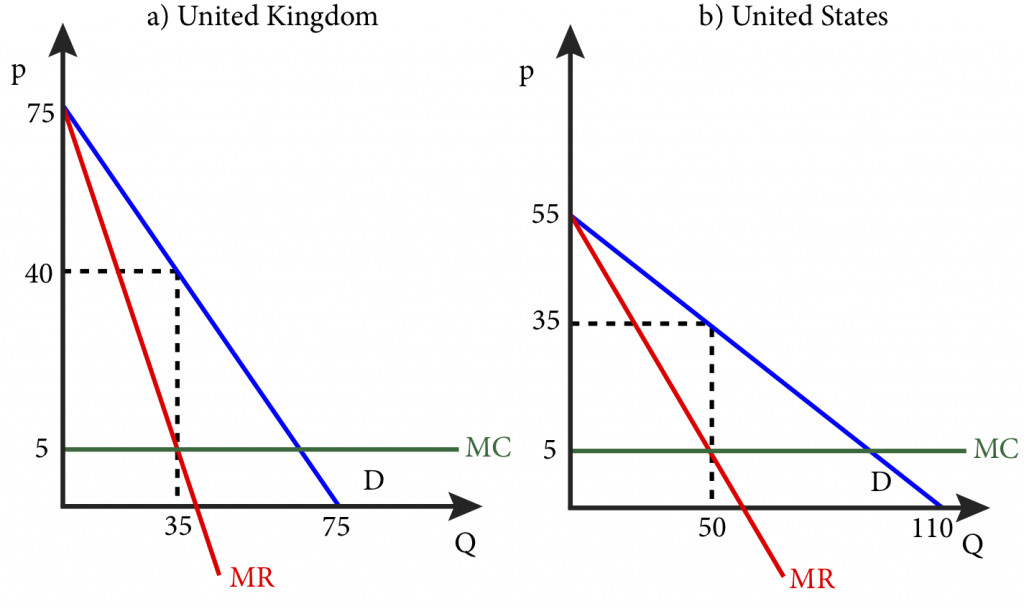
The firm’s producer surplus is the difference between the price of the book and the marginal cost of producing the book multiplied by the number of books sold. The producer surplus in the U.K. is
How much more producer surplus did the firm earn from practicing group price discrimination? We can answer this question by comparing this outcome to the outcome for a simple monopolist that charged the same price for the book in both markets. To figure out the profit maximizing price for the combined market, we have to sum the two demands together. Note that for prices above $55, only U.K. consumers will purchase the book. So let’s begin by assuming that the final price will be below $55 and then we can check this assumption at the end.
Adding to two demands together for prices below $55 begins with adding the quantities together:
\begin{aligned}[t]
Q^{UK}=75-p^{UK}\\
+ Q^{US}=110-2p^{US}
\end{aligned}\\
\begin{aligned}[t]
Q^{TOTAL}=185-3p
\end{aligned}
\end{align}
Putting this into inverse demand format yields:
[latex]p=61\frac{2}{3}-\frac{1}{3}Q^{TOTAL}[/latex]
Giving us a marginal revenue curve of
Setting MR=MC yields:
[latex]56\frac{2}{3}=\frac{2}{3}Q^{TOTAL}[/latex].
Solving this gives us:
We see here that our assumption that the price would be less than $55 is confirmed so consumers in both markets will purchase the book.
The producer surplus in this combined market is thus [latex]PS^{TOTAL}=(33.33-5)×85,000=[/latex]2,408,050 which is almost $67,000 less than the price discriminating monopolist. From this example we can clearly see how offering different prices to the two sets of consumers can improve the outcomes for firms with market power.
15.4 Quantity Discounts or Second-Degree Price Discrimination
Learning Objective 15.4: Describe second-degree price discrimination and how it overcomes the identification problem.
Firm might know that their customers have different demands but they are unable to tell anything about individual demands and are unable to divide them into identifiable groups. However, they might still be able to price discriminate by offering quantity discounts. The key to this type of price discrimination is to offer pricing schemes such that the different types of consumers sort themselves by choosing different deals. Done well, firms can improve profits through the use of such a scheme, and the prevalence of volume discounts in markets suggests that this is a very effective profit-increasing tool for firms with market power.
The effect of volume discounts is to entice high demand consumers to purchase more of the good. This increases overall sales for the producer, which improves their profit. High demanders are better off as well since the opportunity to purchase the output at the normal price is available to them but they choose the volume discount. This type of pricing has another name in economics, non-linear pricing, and is very common in consumer products. To understand the name, consider a linear relationship in the pricing of soft drinks. If a 10oz soda sells for $1, a 15oz soda sells for $1.50 and a 20oz soda sells for $2, there is a linear relationship between the amount of the good and the price. In each case the price per ounce is $0.10. Typically however, we see soda prices that are non-linear. A 10oz soda might be priced at $1 but then the 15oz soda might be $1.25 and a 20oz $1.45, so the price per ounce is declining as the volume increases.
Consider the example of a college convenience store that sells soft drinks from a soda fountain and has a monopoly on soft drink sales on campus. Suppose it knows that there are two types of consumers for its soft drinks: high and low. Low demanders are less thirsty and have an inverse demand curve of [latex]p^{L}=.25-\frac{qL}{100}[/latex] where q is measured in ounces of soda. High demanders are more thirsty and have an inverse demand curve of [latex]p^{H}=.35-\frac{q^{H}}{100}[/latex]. Though the manager of the store knows that these two types of consumer exist, the store has no way of knowing which type a consumer is when they come into the store. The manager also knows that 1/5 of the consumers on campus are of the high type. The manger also knows that each ounce of soft drink costs $0.05 to provide, or the marginal cost of an ounce of drink is $0.05 and there are no fixed costs.
Let’s begin the analysis by asking what the manager would do if she was able to both tell the two types apart and charge them different prices – in other words act as a simple monopolist for both types. Because these are linear demands the marginal revenue of the both have the same vertical intercept, but twice the slope, thus
|
Low Demanders |
High Demanders |
|
[latex]MR^{L}=.25-\frac{q^{L}}{50}=.05=MC[/latex]
|
[latex]MR^{H}=.35-\frac{q^{H}}{50}=.05=MC[/latex]
|
|
QML=10 |
QMH=15 |
|
pML=$0.15 |
pMH=$0.20
|
|
|
|
In other words, the store would sell a 10oz soft drink to low demanders for $1.50 and a 15oz soft drink to high demanders for $3. The profit per customer is $1.00 for the low demanders and $2.25 for the high demanders. This is found by noticing that the per ounce profit is the difference between the prince and the marginal cost, and then multiplying this by the amount each type purchases. Since 4/5 of the customers are low types and 1/5 are high types, the average profit per customer is (4/5)*($1.00)+(1/5)*($2.25) = $1.35
If the stores are unable to tell the two types apart, they could charge the single monopolist price for the low demanders since the high demanders will purchase at that price but the low demanders will not purchase at the high demander monopolist price. This would yield an average per-customer profit of $1.00 as all customers would buy a 10oz. soft drink for $1.50. Alternatively, if they only offered the large drink, only high demanders would buy and though they would make $2.25 in profit on those sales, only 1/5 of the customers will buy so the average profit per customer would be $0.45. It is also immediately clear that if the store offered both deals, no one would buy a 15oz. soda at $3 as for the same price they could buy two 10oz. soft drinks or 20oz. of soft drink.
But what if the store offered a volume discount on soft drink, could they get the high demanders to voluntarily switch to a larger drink and make more money in the process? Consider the following deal: anyone can buy a 10oz. soft drink for $1.50, or a 20oz. soda for $2.40. This second offer is a volume discount as the price per ounce has dropped from $0.15 in the 10oz. case to $0.12 in the 20oz. case. But would this work? Well, low types would only buy 20oz. of the price per ounce is $0.05 so they would choose the 10oz. soft drink. What would high types do? Well, 10oz. of soft drink at a price of $1.50 leaves the high type with $1.50 of consumer surplus as can be seen in Figure 16.4.1 as the green area above the price and below the demand curve. 20oz. of soft drink for $2.40 leaves high demanders with $2.60 in consumer surplus or the green area plus the areas A and C. In other words the large soft drink returns more value to the high demand customers so they will voluntarily choose the larger soft drink while the low demanders will voluntarily choose the smaller soft drink.
Figure 15.4.1: Volume Discounts with Two Types of Consumers
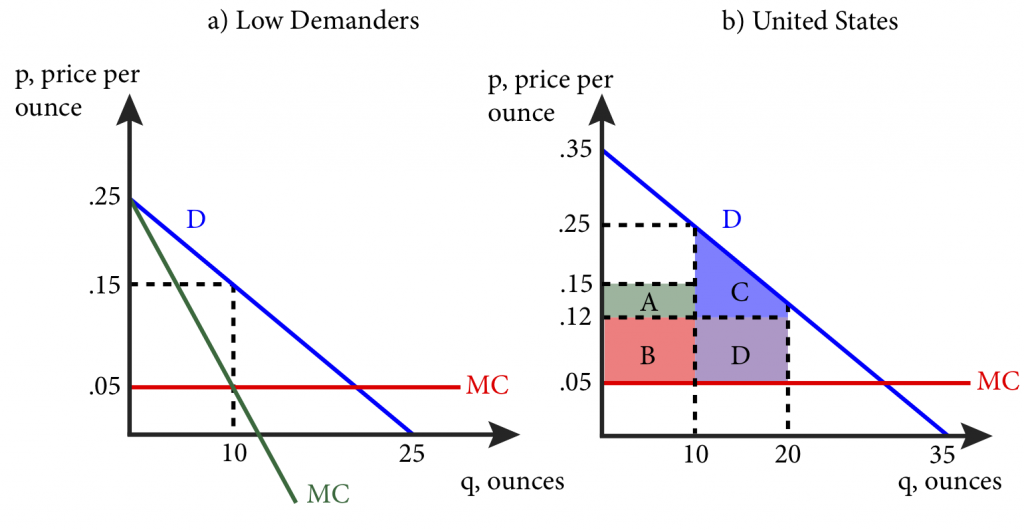
All that remains to be checked is whether it is better for the store to offer the two prices. If they only offered one, we have seen that it is best to offer the 10oz. soft drink for $1.50 and make $1.00 in profit per consumer. With this pricing scheme, all low demanders will buy the 10oz. soda and high demanders will buy the large drink. The per customer profit on the large drink is $1.40, as can be seen in Figure 15.4.1 as areas B and D. So getting the 20% of consumer to voluntarily choose the large drink increases average profit by $0.08. Graphically, this is represented in panel b of Figure 15.4.1 where the store gains area D in profits and gives up area A. Since D is bigger than A, this represents an increase in profits for the store.
This example illustrates how firms with market power who serve customers with different demands can extract more surplus from the market by offering volume discounts which the high demand customers will choose voluntarily.
15.5 Two-Part Tariffs and Tie-In Sales
Learning Objective 15.5: Define two-part tariffs and tie-in sales and how they work as price discrimination mechanisms.
There are other ways that a firm with market power can practice second-degree price discrimination other than quantity discounts. One way is through the use of two-part pricing and the other is through tie-in sales. In both cases the key characteristic is the inability of the producer to identify consumers’ willing ness to pay either as individuals or in groups. Both pricing schemes rely on consumers voluntarily choosing a price based on their demands.
A Two-Part Tariff is a pricing scheme where a consumer pays a lump-sum fee for the right to purchase unlimited number of goods at a unit price. One example of a two-part tariff is a nightclub that charges a cover fee to enter the establishment and, once inside, patrons are able to purchase drinks at set prices. Another example are membership retailers like Costco and Sam’s Club which require patrons to purchase annual memberships to shop at the stores. Two-part tariffs are particularly relevant in the case of multiple purchases as consumers who only purchase one unit are essentially paying a single price but those that purchase more units are lowering their per-unit price as the one-time fee is divided across more units.
To understand how a two-part tariff works, we begin by assuming identical consumers, after examining the identical consumer case, we turn to the case of two types of consumers, high-demand and low-demand to understand how two-part tariffs can work as second-degree price discrimination mechanisms.
Two-Part Tariff with Identical Consumers
Suppose a monopolist knows the demand curve of each of its consumers and that its consumer all have identical demands. For example, suppose the only fitness club in a town knows that every single customer has exactly the same monthly inverse demand curve for visits to the club of p = 10 – ½ q where q is the individual’s quantity of visits. This fitness club is considering using a two-part pricing scheme where it charges a monthly membership fee and a price per-use. Furthermore the club estimates its marginal cost each time a client uses the club at $4. This includes the wear and tear on machines, cleaning, cost of towels and water in the locker room, etc. Since the monopolist knows the demand curves of its customers they can use the per visit price to maximize consumer surplus and then use the monthly fee to extract the entire consumer surplus.
The fitness club’s pricing strategy is illustrated in Figure 15.5.1. By setting the per-use price equal to marginal cost of $4, consumers will choose to visit the fitness club 16 times per month. The total consumer surplus generated from being able to visit the fitness club 16 times in a month at a price of $4 is $48. So if the club charges a monthly membership fee of $48 on top of a per use price of $4, consumers will be willing to pay it as they get $48 of benefit from being able to use the club at $4 a time. The club is able to maximize and extract the entire surplus in the market, identical to a first-degree price discriminator.
Figure 15.5.1: Two-Part Tariff with Identical Consumers
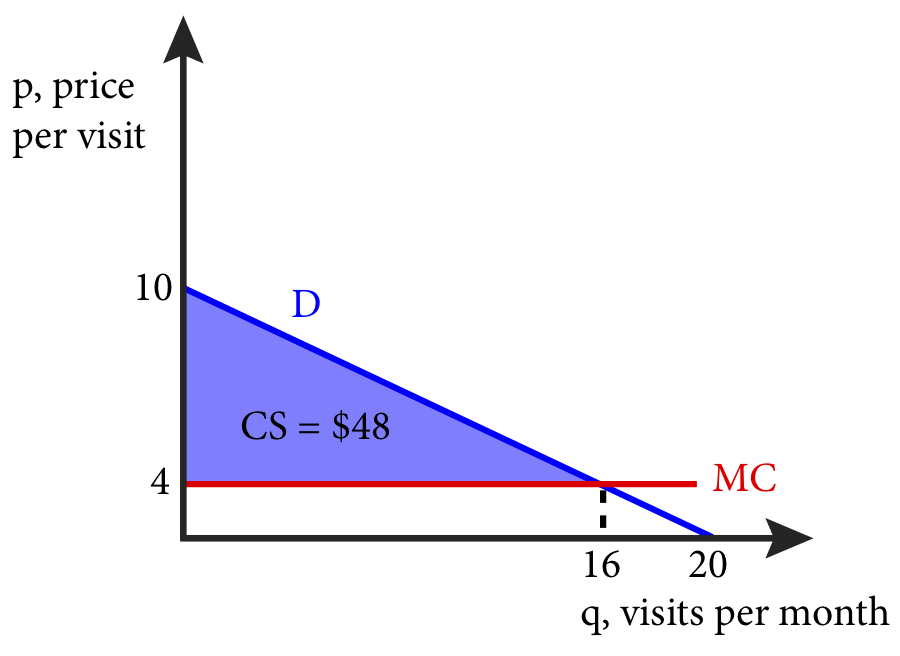
Note that this outcome extracts the maximum surplus possible from the market and the quantity is the same as the perfectly competitive outcome. There is no possible way for a firm to do better than this. It is also a quite simple pricing mechanism but a very effective one.
Two-part pricing with two types of customers
Now let’s explore how a firm, that knows it has different types of consumers but can’t tell them apart, can use two-part tariffs to price discriminate and improve profits. Let’s return to our fitness club but now let’s assume that there are two types of customers: fitness nuts and casual exercisers. Fitness nuts have an inverse demand curve of pH = 24 – qH while casual exercisers have an inverse demand curve that is the same as the previous example: pL = 10 – ½ qL. How can the fitness club use a two-part tariff to practice second-degree price discrimination? Consider offering the same prices as in the previous example but add one further restriction: a monthly membership costs $48, but it entitles the purchaser up to 16 visits in a month for $4 each. We know that the casual exercisers will choose to purchase this as they would choose exactly 16 visits and gain exactly $48 in consumer surplus from consuming 16 visits at $4 each.
Figure 15.5.2: Two-Part Tariff for High Demanders
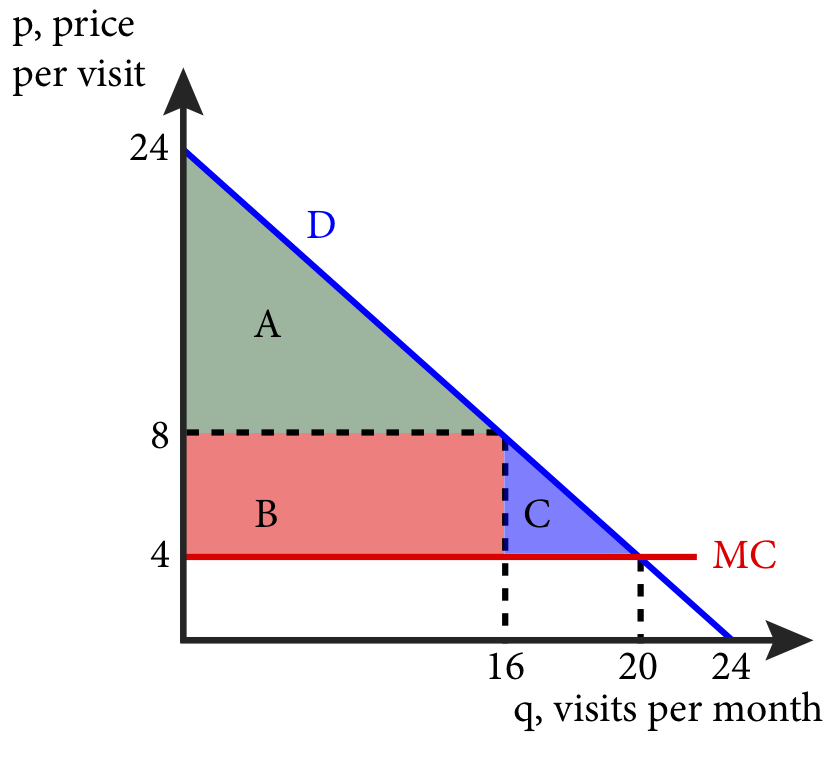
But what about the fitness nuts whose demand curve is shown in Figure 15.5.2? This deal, let’s call it the Silver Membership – 16 visits at $4 each – would yield a total of $192 in consumer surplus (areas A + B), $48 of which would be paid as the monthly membership, netting $144 in leftover consumer surplus that the fitness nuts enjoy. However, note that the fitness nuts would like to visit the club more than 16 times in a month at the price of $4, they would like to visit 20 times. If they were allowed to visit 20 times, they would get a total of $200 in surplus (areas A + B + C). So the club could offer a Gold Membership, a package to more frequent visitors in addition to Silver Membership. If they offered a package that included 20 visits a month, how much could they charge for this enhanced membership? In order to get the fitness nuts to voluntarily choose the Gold Membership over the Silver Membership they need to leave them with as much consumer surplus as the Silver Membership leaves: $144. So $200 – $144 = $56. If the club charged $56 for the Gold Membership, the fitness nuts would voluntarily choose this package. What about casual exercisers? They would not visit more than 16 times in a month even if they had the right to, and they would not generate more than $48 in consumer surplus so a membership of $56 is too expensive and they would not choose it.
This pricing scheme is successful in getting the different types of consumer to self select into different pricing schemes, but is it better for the club? The answer is yes. The club breaks even at every visit since the price of each visit, $4, is exactly equal to the marginal cost of the visit. So by offering Gold Memberships, they collect $56 from types that they would otherwise have earned $48 from. This $8 difference is the improvement in profits for the club. Note that, in this case, the Gold Membership represents a volume discount, as the average cost per-visit for the Silver members is $7, while the average cost per-visit for the Gold members is $6.80.
Now that we have seen how two-part tariffs can be used to increase firm profits and act as a type of price discrimination, let’s consider the practice of tie-in sales.
Tie-In Sales
Tie-in sales refers to situations where the purchase of one item commits consumer to buy another product as well. A very common example is ink-jet computer printers. The printers themselves are sold in quite competitive markets, but the printer requires that only the manufacturer’s ink can be used in the printer creating a situation where the firm has market power in the ink market. In many ways such programs are very similar to two-part tariffs where the price of the printer is like the fixed fee and the ink is the per-use price. However, in tie-in sales it is the printer that is priced competitively and the ink for which a monopoly price is charged.
Other examples include razors and blades, automobiles and ‘genuine’ parts required to keep warranty valid.
15.6 Bundling, Versioning and Hurdles
Learning Objective 15.6: Define bundling, versioning and hurdles and how each works to increase firm profits.
Selling more than one good together for a single price is called bundling. Firms use bundling as another pricing strategy commonly used by firms to increase profit. Pure bundling is when the goods are only sold together at a single price and mixed bundling is when goods are available separately at individual prices and together at a single price that is typically lower than the sum of the two individual prices. Bundling is an alternative pricing strategy that is similar to quantity discounts but generally used in markets for goods where consumers don’t generally purchase more than one units of each at a single time and therefore quantity discounts are not effective. Bundling does require that resale preventable or impractical.
A good example of bundling is cable and satellite television. Most companies sell television channels in packages of channels so, for example, if you are sports fan that wants ESPN you might be forced to choose a package of channels that includes the Home and Garden Network (HGN) which you might not values very highly. On the other hand, you might be very interested in home improvement and value the HGN channel very highly and have a low value for ESPN. By selling them together as a bundle the cable or satellite television provider can improve its profits relative to selling them separately.
To see this consider the following simple example of an economy in which there are two types of television watchers: the sports fans and the home improvers. We will assume that there are equal numbers of both in the economy and that there is a single cable company that provides the channels but the cable provider cannot tell the two types of customers apart prior to a sale. We also assume that the cable company’s marginal cost of an extra subscriber for each channel is zero.
Sports fans like to watch sports and are not very interested in home improvement. Home improvers like to watch home improvement shows and are not very interested in sports. Their reservation prices for a month of access to ESPN and HGN are given in the table below:
Table 15.6.1: Reservation Prices for Television Channels per Month
|
|
Consumer Types |
|
|
Television Channels |
Sports Fan |
Home Improver |
|
ESPN |
$30 |
$12 |
|
HGN |
$14 |
$36 |
|
Both |
$44 |
$48 |
Given this information, what is the best pricing strategy for the cable monopolist? One strategy is to sell the channels separately a la carte style. If they did so, they could charge the monopoly price for each channel. In the case above, the profit-maximizing price for ESPN is $30. Since marginal cost is zero, the profit-maximizing price is the same as the price that maximizes revenue. If the company charges at $30 the sports fan will purchase it, the home improver will not so total revenue is $30 per customer pair. If instead the company charged $12, both consumers would purchase the channel so total revenue would be $24. So $30 is the profit-maximizing price for ESPN. Similarly if the cable company charged $36 for HGN, only home improvers would buy and the revenue per consumer pair would be $36. If they charged $14 both consumers would buy the channel and they would generate $28 per pair. In total the maximum profit per consumer pair from selling the channels separately is $30 + $36 or $66.
Now suppose they sold the two channels only as a bundle, what is the profit maximizing price fort the bundle? If the cable company charged $48 for the bundle, home improvers would buy, sports fans would not and revenue per consumer pair would be $48. If the cable company charged $44 for the bundle, both types would buy and revenues would be $44 × 2 or $88. So clearly the profit-maximizing price for the bundle is $44.
Comparing the bundle revenues to the single channel revenues, it is clear that bundling is a better choice for the company as they earn $88 per consumer pair when they bundle versus $66 when they sell the channels separately. Why is this? By selling them separately their incentive is to charge prices for individual channels that excludes the type that doesn’t prefer the channel. By bundling, the company both forces the consumer to purchase the other, less preferred channel, but offers a substantial discount for the second channel. This gets consumers to buy twice as many channels as they would otherwise which is good for the firm because, at a marginal cost of zero, any extra sales that brings in any additional marginal revenue is profit enhancing.
But bundling does not always result in higher profits. Consider the same example with different reservation prices:
Table 15.6.2: Reservation Prices for Television Channels per Month
|
|
Consumer Types |
|
|
Television Channels |
Sports Fan |
Home Improver |
|
ESPN |
$30 |
$14 |
|
HGN |
$16 |
$10 |
|
Both |
$46 |
$24 |
In this example the sports fan still has a higher reservation price for ESPN and the home improver still has a higher reservation price for HGN, but now the sports fan has relatively high reservation prices for both while the home improver has low reservation prices for both. Now if they charge for the channels separately the profit maximizing prices are $30 for ESPN, which yields $30 in revenue per pair because only the sports fan would purchase it, and $10 for HGN, which yields $20 in revenue per pair, for a total of $50 in revenue. The profit maximizing bundle price is $24, which both consumers would pay and generate a total of $48, or $2 less than selling them separately.
What has changed in these two examples? In the former the reservation prices are negatively correlated across the two types of customers: the sports fan has a higher reservation price for ESPN and a lower reservation price for HGN and the opposite is true for the home improver. In the latter example the reservation prices are positively correlated: the sports fan has higher reservation prices for both channels.
Another form of price discrimination is versioning: the selling of a slightly different version of a product for a different price that does not reflect cost differences. A common example of this is the sales of luxury versions of family sedans by major car companies. The Honda Accord, the Toyota Camry and the Ford Fusion are all mid-sized family sedans. All three come in base models, with a standard set of features and luxury versions with additional features such as more luxurious interior materials such as leather seats, more technological components such as adaptive cruise control systems and the like. If the price differential simply reflected the extra cost to the firm of these extra features, there would be no price discrimination, however this is not the case, car companies charge a premium over additional costs. For this to be a profit maximizing strategy it must be the case that customers with low price elasticities self-select into the luxury versions because preference for luxury amenities and low price elasticity are correlated. They pay a higher price than do customers with higher price elasticities. As we saw in section 16.3, being able to charge the low elasticity customers a higher price than high elasticity customers is often a profit improving strategy.
[Example Honda Odyssey tourning v. elite. $1000 more for nav system]
Another price discrimination mechanism is through the use of hurdles: a non-monetary cost a consumer has to pay in order to qualify for a lower price. The most classic hurdle is the redeemable coupon. Consider a grocery store that prints a sheet of coupons and outs them in a flyer in the local newspaper or sends it in the mail. All customers of the store have a chance to use them but many don’t. Those that do pay a price to use them, the time and effort of cutting them, searching for the specific good for each coupon and redeeming them at check out. The price discrimination mechanism is that high elasticity customers are more likely to pay the cost of dealing with coupons and at the same time, these are the very customers to whom the stores would like to offer a lower price.
Other examples of hurdles are early-bird dinner deals, mail-in rebates, matinee movie tickets, paperback versions of popular books and rush tickets for theater performances only available the night of the performance. In all cases there is some cost to purchasing the product – having to arrive early to a restaurant or movie, not being able to be guaranteed a seat at a theater, having to wait to but a book, having to fill-out a rebate card and wait 6-8 weeks for a check – that entitles to the person paying the cost to a lower price. This cost causes consumers to self-select into two groups, those that pay the cost to get a lower price and those that pay the higher price and avoid the cost.
SUMMARY
Review: Topics and Related Learning Outcomes
15.1 Market Power and Price Discrimination
Learning Objective 15.1: Explain differentiated pricing and describe the three types of price discrimination.
15.2 Perfect or First-Degree Price Discrimination
Learning Objective 15.2: Describe first-degree price discrimination and the challenges that make it hard.
15.3 Group Price Discrimination or Third-Degree Price Discrimination
Learning Objective 15.3: Describe third-degree price discrimination and its effect on profits.
15.4 Quantity Discounts or Second-Degree Price Discrimination
Learning Objective 15.4: Describe second-degree price discrimination and how it overcomes the identification problem.
15.5 Two-Part Tariffs and Tie-In Sales
Learning Objective 15.5: Define two-part tariffs and tie-in sales and how they work as price discrimination mechanisms.
15.6 Bundling, Versioning and Hurdles
Learning Objective 15.6: Define bundling, versioning and hurdles and how each works to increase firm profits.
Learn: Key Terms and Graphs
Terms
Graphs
First-Degree Price Discrimination
Group or Third-Degree Price Discrimination in Book Publishing
Volume Discounts with Two Types of Consumers
Two-Part Tariff with Identical Consumers
Two-Part Tariff for High Demanders
Tables
Reservation Prices for Television Channels per Month
Reservation Prices for Television Channels per Month
Supplemental Resources
Practice Questions
YouTube Videos
There are no supplementary YouTube videos for this module.
Policy Example
Policy Example: Should Public Universities Charge Everyone the Same Price?
Learning Objective: Explain how the use of price discrimination can be seen as a way for public universities to accomplish their mission.
The public universities of the United States, like the University of California at Berkeley have a common foundational purpose: to provide a quality education for the students of the state in which they reside. To provide a quality education comes at a considerable cost, as universities are complex institutions that house, feed and educate students. But is price discrimination antithetical to their mission? As non-profit entities, what motivates universities to charge such different prices if profit is the motivation for price discrimination of most companies?
To understand the rationale behind the use of price discrimination lets begin be thinking of a demand curve for a local public state university. There are a limited number of places the university can offer each year and a selection process so let’s think of the demand curve for accepted students, those that have ben offered a spot. The demand curve for these admitted students is very likely downward sloping as there are probably only a few families that could potentially afford a very high tuition but as tuition declines more and more families are able to and would choose to purchase a college education at the institution. There is also a competitive effect as there are other colleges and universities competing for the same students but let’s deliberately ignore that to focus on the questions at hand. We will also assume the marginal cost of each student is constant. The key to understanding the university’s situation is to think about what the excess revenues above marginal cost represent. As a not-for-profit institution we can think of this as going toward paying the fixed costs of the school, much of which is represented by the tenured faculty and the research and teaching infrastructure, which we could loosely call the quality component. More or better professors, better labs, classrooms, etc. both cost more and contribute to the quality of the education.
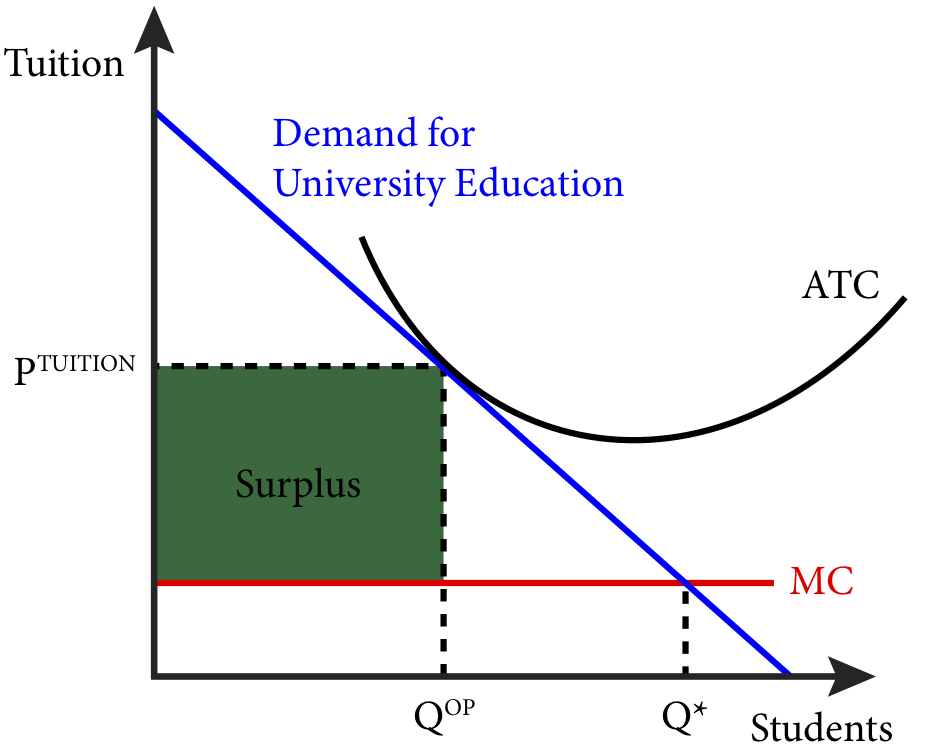
A university has a number of options when thinking about the price to charge for tuition. Because they can both charge a price to each individual and, by collecting detailed information on family finances, they can tailor that price to their ability to pay they can overcome the two challenges to price discrimination – preventing arbitrage and acquiring information. So universities can engage in very sophisticated pricing strategies. But should they. One option is to charge a single price that allows them to break even by exactly covering their total costs. This solution is seen in Figure 1. The tuition price, PTUITION is such that at the quantity demanded at the price, QOP, the total revenue equals the total cost. In this scenario, QOP students attend the school and all pay exactly the same price.
Figure 1: Universities Charging a Single Price
If instead the university practices price discrimination, they can charge each student exactly their reservation price. This situation is illustrated in Figure 2.
Figure 2: Universities Practicing First-Degree Price Discrimination
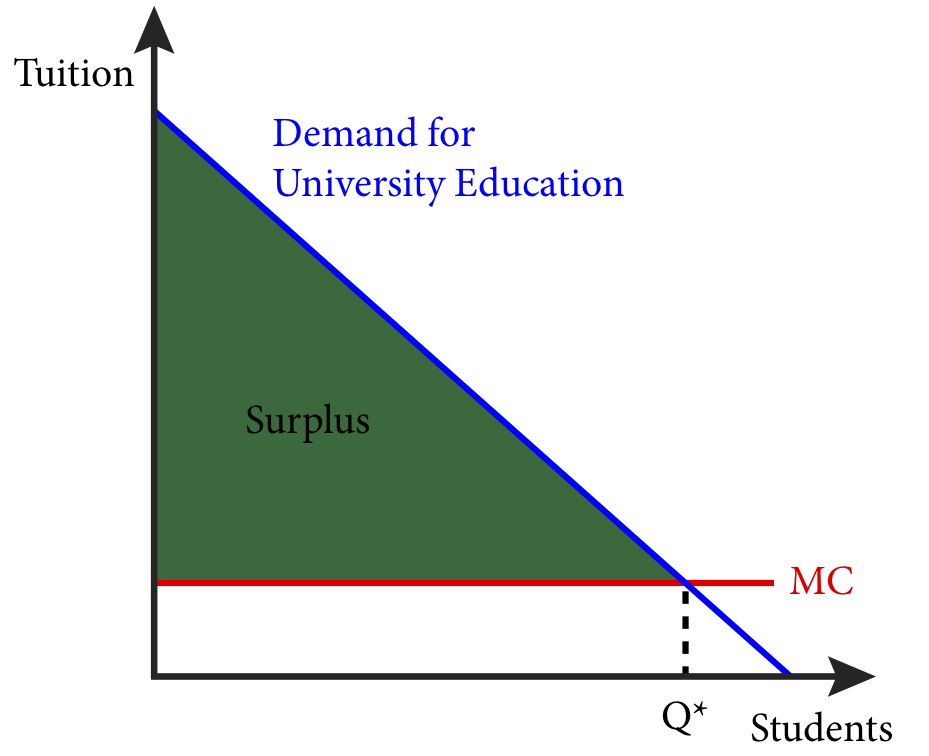
Because the university can charge individual prices, they will continue to offer tuition prices to all students whose reservation price is at least equal to marginal cost. This means that they will end up serving Q* students. This is substantially more students than the single price strategy, and is also the socially optimal number of students. In this case, the university captures the entire area beneath the demand curve and above the marginal cost as producer surplus. This is also substantially more than the producer surplus in the one price scenario and is money that the university can invest in improving the quality of the school.
By practicing very sophisticated price discrimination, the university comes close to the first-degree price discriminator’s outcome where the number of students is the socially optimal Q*, but the school captures all of the surplus for itself to cover fixed costs and invest in quality. So from an institutional level we could argue that this is a good thing. What about for individual students? The answer depends on where on the demand curve the individual students lie. For wealthier students or those that otherwise had a very high reservation price, they are playing quite a bit more than they would with a single price. But for low income or otherwise low reservation price students, with price discrimination that can attend the university where otherwise they would have been shut out with a single price and they pay a lower tuition than the single price.
Exploring the Policy Question
- Explain how high income students might feel very differently about the price discrimination by state universities than low-income students.
- Do you think that price discrimination is consistent with the mission of your school?
- How do you feel about the price you play for college – is it fair?
Candela Citations
- Authored by: Joel Bruneau & Clinton Mahoney. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike
- Module 16: Pricing Strategies. Authored by: Patrick Emerson. Retrieved from: https://open.oregonstate.education/intermediatemicroeconomics/chapter/module-16/. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike
- Price Discrimination. Authored by: Preston McAfee & Tracy R Lewis. Retrieved from: https://resources.saylor.org/wwwresources/archived/site/textbooks/Introduction%20to%20Economic%20Analysis.pdf. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike
monopolists that are limited to a single price at which all of the output they produce is sold
selling the same good or service for different prices to different consumers
the practice of charging different prices for the same good to different consumers
or first-degree price discrimination, is a type of pricing strategy that charges every consumer a price equal to his or her willingness-to-pay
or third-degree price discrimination, is charging different prices for the same good or service to different groups or different types of people
or second-degree price discrimination, is when firms charge a lower price per unit to consumers who purchase larger amounts of the good
the economic costs of buying and selling a good or service beyond the price itself
exists when a firm charges different per unit prices based on volume; for example one pound of meat may cost $5 to purchase, whereas two pounds may cost $9
is a pricing scheme where a consumer pays a lump-sum fee for the right to purchase unlimited number of goods at a unit price
refers to situations where the purchase of one item commits consumer to buy another product as well
selling more than one good together for a single price
is when two or more goods are only sold together at a single price
is when goods are available separately at individual prices and together at a single price that is typically lower than the sum of the two individual prices
the selling of a slightly different version of a product for a different price that does not reflect cost differences
a non-monetary cost a consumer has to pay in order to qualify for a lower price

